ACCIDENTAL NECESSITY AND POWER OVER THE PAST

The thesis of this paper is that an agent S has the power to bring it about that a proposition p is or will be true at a moment t only if S has at the same time the power to bring it about that it has always been the case that p would be true at t. The author first constructs a prima facie compelling argument for logical determinism and then argues that whoever accepts an Ockhamistic response to that argument should also accept the above thesis.

Some philosophers have recently defended what is commonly regarded as a startling and even obviously false claim, viz. that it is at least conceivable that some agent have the power to bring about the past.1 But this claim is actually much too modest, since, as I hope to show, we have the sort of power that we ordinarily attribute to ourselves only if we in fact have power over the past as well. More precisely, the thesis I wish to defend is that an agent S has the power to bring it about that a proposition p is or will be true at a moment t only if S has at the same time the power to bring it about that it has always been the case that p would be true at t. One noteworthy corollary of this thesis is that the power to bring about the past is so commonplace that such power would be relatively uninteresting if it were not so frequently claimed to be impossible.

Since the sort of power I have in mind is rather mundane, this paper will not include any discussion of such exotic items as time travel, deviant notions of causality, or precognitive beings whose present actions are influenced by what they know other agents will do in the future. Instead, I will set the stage for my main argument by examining in some detail the familiar but often misunderstood claim that the past is unalterable. This will lead to a brief examination of a prima facie compelling argument for logical determinism and of what I take to be the only three interesting lines of response to that argument. Then I will argue that anyone who accepts the Ockhamistic line of response, which in my view is the most attractive, should also accept the thesis that we have power over the past.

It is by no means a simple task to capture in a general formula our intuitions about the unalterability of the past. Consider the following principle, which has appeared in the philosophical literature:2 /55/

(A) No one has the power at any moment t to do anything such that were he to do it, what is at t a fact about the past would at some time after t no longer be a fact about the past.

(A) is initially attractive. For instance, it is reasonable to believe that since it is now a fact about the past that Caesar crossed the Rubicon, no one now has the power to bring it about that tomorrow it will no longer be a fact about the past that Caesar crossed the Rubicon. But while few would challenge this example, (A) has other consequences which are less benign. Suppose, for instance, that it is now true that David has never been to Chicago. This is clearly a fact about the past. Now no one would object to the further prosaic assumption that David now has the power to do something (e.g., board an airplane) which will result in his being in Chicago two hours from now. Yet (A) renders this assumption impossible. For if David were now to exercise the power in question, then two hours from now it would no longer be a fact about the past that David has never been to Chicago.

So (A) is unacceptable as it stands. But at this point it would be natural to suggest the following modification:

(A*) No one has the power at any moment t to do anything such that were he to do it, what was a fact at an earlier moment t* would not have been a fact at t*.

(A*) seems invulnerable to the above counterexample. If it was a fact yesterday that David had never been to Chicago, no one can now bring it about that it was not a fact yesterday that David had never been to Chicago.

However, (A*) is ambiguous. On one reading, it is simply a consequence of the more general thesis that no agent has the power to make both a proposition and its negation true at the same moment. So no one can have the power at a moment t to bring it about that what was true at an earlier moment t* was also not true at t*. While (A*) is undoubtedly true on this reading, it does not give us what we were looking for, viz. a general principle which captures the asymmetry between our control over the past and our control over the future. For it is equally true, as many have pointed out, that no one can have the power at t to bring it about that what will be true at a later moment t* will also not be true at t*. So on this first reading, (A*) is true but not to the point.

However, the second--and, I take it, the more natural--reading is more interesting. Consider the following proposition:

(1) Caesar is crossing the Rubicon was once true.

If we let "P" stand for the past-tense propositional operator, then (1) is logically equivalent to

(2) P (Caesar is crossing the Rubicon).

On the second reading, (A*) presupposes that if (2) is now true, then it can no longer be false. That is, if (2) is true now, then it is also in some sense necessary now as well. Consequently, an agent S can now have the power to make the negation of (2) true, i.e., to make it true that Caesar never crossed the Rubicon, only if S can also bring it about that at some past moment both the proposition Caesar is crossing the Rubicon and its negation were true. So some true logically contingent propositions (e.g., (2)) are such that their negations not only are not /56/ in fact true now, but cannot be true now. But now consider the following proposition:

(3) David is in Chicago will be true at some time.

If we let "F" stand for the future-tense propositional operator, then (3) is logically equivalent to

(4) F (David is in Chicago).

Suppose that (4) is now true. It does not follow from (A*), on the second reading, that (4) now has the same modal status as (2). Nor does there seem to be any other plausible general principle from which this would follow. (4) is, after all, a fact about the future and not about the past. Hence, at least prima facie it seems that (A*) is consistent with the claim that even though (4) is now true, it is not now necessary in the way in which (2) is. And so it appears that on the second reading, (A*) does indeed point to the asymmetry we were trying to capture. So (A*), taken on this second reading, is the principle we were looking for--or so, at least, it seems. (When I refer to (A*) hereafter, I will, unless otherwise indicated, have the second reading in mind.)

Nevertheless, there are, I will argue, weighty reasons for rejecting (A*). For (A*) is a consequence of and is epistemically supported by a principle which is arguably the weakest link in a prima facie compelling argument for logical determinism. And once we reject this principle, we open the door to a strong positive argument for the conclusion that we do indeed have power over the past.

(A*) is unduly inexplicit about the sort of necessity which now attaches to (2) in virtue of its being a fact about the past. The necessity in question is clearly not logical (i.e., metaphysical), physical or causal necessity.3 Rather, it is a necessity which, to put it crudely, is had by the past simply because it is the past. Medieval logicians called this modality necessity per accidens, i.e., accidental necessity. By investigating accidental necessity in some detail, we will be able to come to a deeper understanding of the issues involved in the question of whether we have power over the past.

I will begin by fashioning a framework within which we can talk precisely about the necessity of the past. First, I will make the simplifying (in this context) assumption that all propositions are tensed. Though this assumption seems to me both natural and true, it may not be absolutely crucial. But I will leave it to the friends of "tenseless" propositions to reconstruct what I will say in their own idiom. The assumption in question has two relevant consequences. The first is that some propositions can be true at some times and false at others. Examples are the present-tense proposition David is in Chicago and the past-tense proposition Socrates drank hemlock. Again, the future-tense proposition Socrates will meet with Plato might be true now even though it will be false after Socrates and Plato have met for the last time. The second consequence is that some present-tense propositions can be true at at most one moment. Examples are the propositions David is in Chicago at T and T is present (or: it is (now) T), where T is a single determinate moment of time. Each of these propositions can be true only at T. /57/

I will now mention a few of the basic properties of accidental or per accidens modality. First, since a proposition which is necessary per accidens is, as the name suggests, such that its being necessary is an accidental feature of it, only logically contingent propositions can be necessary per accidens. An analogous point holds for the mode of accidental impossibility. So in this regard per accidens modality resembles physical and causal modality.

Second, a proposition's being necessary per accidens is, as should be expected, relative to a time. For a proposition can--and typically does--become necessary per accidens after not having been necessary. For instance, the past-tense proposition Socrates drank hemlock is now (presumably) necessary per accidens and thus can no longer be false. But it was false when Socrates was a child. Again, an analogous point holds for accidental impossibility.

From these first two points it follows that for any moment t, logically contingent propositions may be divided into three mutually exclusive and jointly exhaustive groups: (a) those which are necessary per accidens at t; (b) those which are impossible per accidens at t; and (c) those which are neither necessary per accidens at t nor impossible per accidens at t. I will say that the members of this third group are "temporally contingent" at t.

Third, a proposition's being necessary (impossible) per accidens at a given moment entails that it remains necessary (impossible) per accidens at every succeeding moment. This, again, is what we should expect to be true of the necessity of the past. Also, since it appears to be logically possible for a proposition to be physically necessary (or a law of nature) at one moment and not at some later moment, this feature of accidental necessity distinguishes it from both physical and causal necessity. So some logically contingent propositions, e.g. (presumably), Socrates drank hemlock, are not now and never will be possibly false, and their negations are not now and never will be possibly true, where the impossibility in question is accidental impossibility. Moreover, from this it follows that if p is necessary per accidens at t, then no one has the power at or after t to bring it about that p is or will be false. And it also follows that if p is impossible per accidens at t, then no one has the power at or after t to bring it about that p is or will be true. In short, the necessity of the past entails its unalterability. So the contention that no one can ever have the power to alter a fact about the past is acceptable only if we are careful to interpret it as follows: no proposition which is now necessary per accidens is now or ever will be such that some agent has the power to make it false, and no proposition which is now impossible per accidens is now or ever will be such that some agent has the power to make it true. And this is so even if the agent in question is omnipotent.4

Fourth, when we limit the consequents to logically contingent propositions, accidental necessity, like other kinds of necessity, is closed under entailment.5 That is,

(B) If p entails q, and q is logically contingent, and p is necessary per accidens at t, then q is necessary per accidens at t.

Further, given what was said in the preceding paragraph, it should be clear that the conjunction of (B) with the obvious truth that no one has the power to make a logically necessary proposition false entails /58/

(C) If p entails q, and p is necessary per accidens at t, then no one has the power at or after t to bring it about that q is or will be false.

(C), it should be evident, is beyond dispute. If p cannot be false at or after t, then no proposition entailed by p can be false at or after t--and so no one has the power at or after t to make such a proposition false. Likewise, it is easy to show that if p is impossible per accidens at t, then no proposition which entails p can be true at or after t--and no one has the power at or after t to make such a proposition true.

We now have a general framework for discussing the necessity of the past. The obvious next step is to address the substantive question: just which propositions are necessary or impossible per accidens at a given moment? Let "P" and "F" stand, as before, for tense operators, and let "N" stand for the negation operator. Philosophers from Aristotle in ancient times to Arthur Prior in our own century have, at least implicitly, accepted the following principle:

(D) If p is true at t, then Pp is necessary per accidens at every moment after t, and NPp is impossible per accidens at every moment after t.

Once a proposition p has been true, it is always necessarily true afterwards that p was once true. And once p has been true, it is always necessarily false afterwards that p has never been true. So not only is it the case that it will never in fact be false after t that p was true, but it is also the case that it cannot be false after t that p was true. According to the proponent of (D), this amounts to saying, in possible worlds jargon, that in every possible world just like ours up to and including t, it is true at every moment after t that p was true. So, given (D), the thesis that the past is necessary can be stated as follows: for any proposition p, if it is now the case that p has been true, then it is also necessary per accidens that p has been true; and if it is now not the case that p has never been true, then it is also impossible per accidens that p has never been true.

A moment's reflection reveals that (D) has (A*) as a direct consequence. For (A*) in effect tells us that once a proposition p has been true, then no one has the power to bring it about that p was never true. Moreover, (D) provides epistemic grounds for accepting (A*), since it explains our lack of power over the past by reference to the necessity of the past. If (D) turned out to be false, it is not clear just what reason we would have for accepting (A*) in all its generality.

Given the popularity enjoyed by (D), it is hard to deny its intuitive appeal. At the very least, there is no obvious alternative on the horizon. However, the disturbing fact is that (C) and (D) provide us with all we need to construct the strongest (and, to my mind, the clearest) possible argument for logical determinism. Let "T" designate some determinate moment of time. The argument can then be formulated as follows:

(P1) F (David is in Chicago at T) is true now, long before T. (assumption)

(P2) So PF (David is in Chicago at T) will be necessary per accidens at every future moment, including every future moment which precedes or is identical with T. (from P1 and (D))

(P3) But PF (David is in Chicago at T) entails If T is present, then David is in Chicago. (assumption) /59/

(P4) Therefore, no one (including David) will have the power at or before T to bring it about that If T is present, then David is in Chicago is or will be false. That is, no one will have the power at or before T to make it true that David is not in Chicago when T is present. (from (P2), (P3) and (C)).

Given (D), the move from (P1) to (P2) is straightforward. Moreover, (P3) simply reflects the normal belief that if it has ever been the case that p will be true at a moment t, then either p has already been true at t, or p is now true (at t), or p will be true at t--depending on whether t is now in our past, our present or our future. In short, if it has ever been the case that p will be true at t, then p is true whenever t is present. But if this is so, then, given (C) , the necessity of the proposition PF (David is in Chicago at T) entails our inability to affect the present and future truth-values of the proposition When T is present, David is in Chicago.

So (C) and (D) enable us to reason validly from the unremarkable assumption that it is now the case that David will be in Chicago at T to the outrageous conclusion that no one will ever have the power to prevent David's being in Chicago at T. (Notice that this is so whether the notion of power is given a libertarian or a compatibilist construal.) Further, the argument is not only valid but perfectly general, since similar deterministic consequences follow whenever we substitute for the proposition F (David is in Chicago at T) any other future-tense proposition whose present-tense counterpart can be true at at most one moment.

Solutions to this argument fall into three main groups. "Aristotelian" solutions all make the drastic move of denying the assumption (P1), claiming either (a) that where p is a future contingent, both p and its negation are neither true nor false; or (b) that where p is contingent, it is false both that p will be true and that its negation will be true, i.e., both Fp and FNp are false. The first of these claims is commonly attributed to Aristotle, while Prior is responsible for the second.6

A second type of solution, at least suggested by some remarks made by Peter Geach, consists in rejecting the assumption (P3) in favor of the rather startling claim that future-tense propositions express only present intentions, dispositions and tendencies.7 So, for instance, even though it might have been true on Monday that David would be in Chicago on Tuesday, it could still be true that David is never in Chicago on Tuesday. For on Monday he may have intended to be in Chicago on Tuesday, but then changed his mind.

"Ockhamistic" solutions, on the other hand, all deny the inference from (P1) to (P2) on the ground that (D), despite its initial attractiveness, is a needlessly and unacceptably strong interpretation of our pre-theoretic beliefs about the necessity of the past. For, according to the Ockhamist, when p is a future-tense proposition true before t, it simply does not follow that in every possible world just like ours prior to t, Pp is true at t and at every moment after t. The most pressing task facing the Ockhamist is to explicate the notion of two worlds being just like one another prior to a given moment in a way which (a) is strong enough to render plausible the claim that two such worlds share the same history at the moment in question and (b) is weak enough not to lead to deterministic consequences when combined with assumptions like (P1) and (P3). This turns out to be a very difficult and complicated task. Roughly speaking, the Ockhamist's /60/ strategy is to find a plausible way to distinguish the propositions which are "presently" true at a given moment t from the past-tense and future-tense propositions true at t. The latter, he holds, are true at t only in virtue of what was "presently" true at moments before t or what will be "presently" true at moments after t. He then substitutes for (D) the weaker claim that a proposition p is necessary per accidens at t just in case p is a logically contingent proposition which is true at every moment at and after t in every possible word which shares all of our world's "presents" prior to t. This invalidates the move from (P1) to (P2), since in some possible world which shares all of our world's "presents" up to and including the present moment, David is not in Chicago at the time in question. So in that world the proposition PF (David is in Chicago at T) is never true, from which it follows that this proposition, though true before T in our world, is never necessary per accidens before T in our world.

In short, the Ockhamist holds that since the present-tense proposition David is in Chicago at T has not yet been true, the past-tense proposition PF (David is in Chicago at T), though now true, does not count as part of our history at the present moment. And, he insists, only someone with implausibly inflated notions of actuality and history would think otherwise. To put it very roughly, then, the Ockhamist contends that the only past-tense propositions which do count as part of our history (and hence are necessary per accidens) are those whose present-tense counterparts have already been true. So he agrees, for instance, that no one can now have the power to make it true that Caesar never crossed the Rubicon. But a relevant corollary of his position is that no argument based simply on the necessity of the past is sufficient to yield the conclusion that if it has already been true that David will be in Chicago at T, then no one can have before T the power to bring it about that the proposition NF (David is in Chicago at T) has been true at every past moment. So in rejecting (D), the Ockhamist casts a shadow of doubt on (A*) as well.

In another place I have defended in some detail an Ockhamistic theory which, I believe, successfully solves the problems that have plagued its historical ancestors.8 However, here I will be content to argue for the conditional thesis that if an Ockhamistic response to the argument for logical determinism is correct, then we in fact have power over the past. Nevertheless, the discussion of logical determinism has strengthened my dialectical position. For of the three types of responses mentioned above, only the Ockhamistic response is compatible with each of the commonly accepted theses (a) that if p is or will be true, then Fp has been true at every past moment, and (b) that if t is present and t is prior to t* and F(p-at-t*) has ever been true, then p will be true at t*. In what follows I will argue that anyone who accepts these theses while rejecting logical determinism is committed to the claim that we have power over the past. So if my argument is successful, then one can deny that we have power over the past only by embracing either logical determinism or one of the first two lines of response described above. My suspicion is that many who are antecedently inclined to deny that we have any power over the past will find each of these alternatives highly implausible. In fact, it is worth noting that no one who espouses causal determinism, whether of the hard or soft variety, can accept either of the first two lines of response mentioned above, since both presuppose that the truth-values /61/ of some future-tense propositions are not now fixed in the way demanded by a thoroughgoing causal determinism. In short, in order to avoid being a logical determinist, a causal determinist has no choice but to accept the Ockhamistic position. So causal determinists constitute a subset---but only a proper subset--of the set of those at whom the following argument is aimed.

It is important to notice that the Ockhamist position does not by itself entail the negation of (A*) or the concomitant thesis that we do in fact have at least some power over the past. In fact, it might appear that the Ockhamist's most prudent strategy is simply to withhold judgment on (A*). However, I will now argue that this appearance is deceptive.

Let K be a linearly ordered set, the set of times. And let T and T* be two members of K such that (a) T > T*, i.e., T* is prior to T, and (b) for some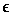 tK, T* > t, i.e., T* is not the first moment of time.9 Further, let "now" be a non-rigid designator indicating the present moment relative to an assignment of truth-values. For instance, given the Ockhamistic position, the future-tense proposition F (David is in Chicago) is true just in case for some t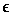K, t > now and David is in Chicago will be true at t. Now consider the following propositions:

(5) David is in Chicago will be true at T, and

(6) F (David is in Chicago at T) was true at every moment prior to T* .

In what follows I will support the claim that we have power over the past by arguing that if an agent S has the power at T* to bring it about that David is in Chicago will be true at T, then S also has the power at T* to bring it about that the future-tense proposition F(David is in Chicago at T) was true at every moment before T*. That is, I will argue that the following conditional proposition is true, where "S" ranges over human agents:

(7) If S has the power at T* to make (5) true at T*, then S has power at T* to make (6) true at T*.

My argument will be generalizable in obvious ways to other propositions and to other pairs of times meeting the two conditions (a) and (b) imposed above on T and T*.

If the Ockhamist is correct, then (6) is temporally contingent at T*, since its truth-value at T* depends on what will be "presently" true at a future moment, viz. T. So, to corroborate a point made above, no Ockhamist can reject (7) on the ground that (6) is either necessary or impossible per accidens at T* and hence such that no one can affect its truth-value at or after T*. Further, I am assuming that any Ockhamist is willing to concede that someone might have the power at T* to make (5) true at T*.10 Of course, this concession entails the claim that the proposition F (David is in Chicago at T) might be true before T. But unlike the Aristotelians, the Ockhamist considers this claim wholly acceptable and perhaps even self-evident. For it follows directly from the first of the two theses alluded to near the end of section I, viz. /62/

(E) If p is or will be true, then Fp has been true at every past moment.

Now it is reasonable to believe that having the power to make (5) true at T* is equivalent to having the power to make the following conjunctive proposition true at T*:

(8) T* = now, and David is in Chicago will be true at T

That is, having the power to make (8) true is tantamount to having the power to make (5) true while T* is present. Nevertheless, someone might object to this claim as follows: "No human agent ever has the power to bring it about that a given moment is present. That is, moments become present or past completely independently of anything we do or can do. But the following principle is obvious:

(F) If S has the power to make p and q true at t, then (i) S has the power to make p true at t and (ii) S has the power to make q true at t.

However, given (F), no agent has the power to make (8) true at T* unless he also has the power to make the proposition T* = now true at T*. Since no agent has this latter power, no agent can make (8) true at T*.

Though (F) is not self-evidently false, this very example should make us suspicious. Moreover, other examples are even more striking. For instance, it seems eminently reasonable to suppose that David, never having been to Chicago, might have the power at T* to make it true that he will someday be in Chicago for the first time. However, if (F) is true, then he cannot have such power. For the supposition in question entails that David has the power at T* to make the following conjunctive proposition true:

(9) David is in Chicago was false at every moment before T*, and David is in Chicago will be true at some moment after T* .

But even the Ockhamist believes that the first conjunct of (9) is necessary per accidens at T* and, hence, that no one has the ability at T* to affect its truth-value. So even power as ordinary as that in question here is ruled out by (F). Again, even a power as commonplace as, say, the power to paint a wooden chair black involves the ability to make certain bodily movements while a given set of physical laws hold, e.g., laws of gravity, laws governing the adhesion of paint to wooden surfaces, etc. So, it seems, to attribute to an agent even so unremarkable a power is to attribute to that agent the power to bring about a complex state of affairs, many of the components of which (e.g., physical laws) are beyond his power to bring about. All of this suggests that we should reject (F) in favor of some version of what Roderick Chisholm calls the "principle of the diffusiveness of power.''11

For present purposes we need only a very weak version of this principle, viz.

(G) If (i) S has the power to make p true at t and (ii) q is or will be true at t and (iii) no agent has ever had or ever will have the power to make q false at t, then S has the power to make p and q true at t.

Given (G) , it follows that someone who has the power to make

(5) David is in Chicago will be true at T

true at T* also has the power to make /63/

(8) T* = now, and David is in Chicago will be true at T

true at T*. For no one has ever had or ever will have the power to bring it about that the proposition T* = now is false at T*.

Moreover, we can explain the intuitive appeal of (F) by pointing out that a principle like (F) is a plausible constraint on any account of just which actions are "basic" for an agent at a given time, where basic actions, in Chisholm's words, "are things we succeed in doing without undertaking still other things to get them done." 12 For if S has the power to make p and q true at t as a basic action,, then it is reasonable to think that S must have the power to make p true at t and also the power to make q true at t. Furthermore, even if (F) is false, the following weaker principle is clearly acceptable:

(H) If S has the power to make p and q true at t, then either (i) S has the power to make p true at t or (ii) S has the power to make q true at t.

It is difficult to imagine what a genuine counterexample to (H) would even look like.13

Now it might appear at this point that the denouement is at hand. For, as we have seen, whoever has the power at T* to make (5) true at T* also has the power at T* to make (8) true at T*. But given the Ockhamistic thesis (E) noted above, (8) entails

(6) F (David is in Chicago at T) was true at every moment prior to T* .

And the following principle seems highly plausible:

(I) If (i) p entails q and (ii) S has the power to make p true at t, then S has the power to make q true at t.

So whoever can make (8) true at T* can also make (6) true at T*. And by the transitivity of implication it follows that whoever has the power to make (5) true at T* also has the power to make (6) true at T* . In short, (7) is true.

Unfortunately, this argument will not work. For, as Joshua Hoffman and Gary Rosenkrantz have recently shown, (I), unlike close analogues for the modes of logical necessity and possibility, is false--even when we limit the range of p and q to logically contingent propositions.14 To put it succinctly, power is not closed under entailment. For instance, the proposition Some rocket ship is red entails the proposition There is a rocket ship. But, obviously, an agent might have the ability to paint a rocket ship red without having the technical expertise required to bring a rocket ship into existence. So (I) is false, and we must look elsewhere for a defense of (7).

Luckily, help is not far off. Even though (8) is not logically equivalent to (6), it is logically equivalent to the following close relative of (6):

(10) T* = now, and F (David is in Chicago at T) was true at every moment prior to T* .

The inference from (8) to (10) is, as above, sanctioned by the Ockhamistic thesis (E). And the inference from (10) to (8) is sanctioned by the second of the two theses noted near the end of section I:

(J) If (i) t = now and (ii) t* > t and (iii) F(p-at-t*) was true at some moment before t, then p will be true at t*. /64/

So (8) and (10) are logically equivalent. But the following principle, unlike its cousin (I), is impeccable:

(K) If (i) p is logically equivalent to q and (ii) S has the power to make p true at t, then S has the power to make q true at t.

Thus, whoever has the power to make (8) true at T* also has the power to make (10) true at T*.15

We now have all we need to construct a valid deductive argument for (7). Suppose that the antecedent of (7) is true. That is, suppose that S has the power at T* to make

(5) David is in Chicago will be true at T

true at T*.

Then it follows from (G) that S also has the power at T* to make

(8) T* = now, and David is in Chicago will be true at T

true at T*. But by the Ockhamistic theses (E) and (J), (8) is logically equivalent to

(10) T* = now, and F (David is in Chicago at T) was true at every moment prior to T* .

So, by (K), S has the power at T* to make (10) true at T* . But S does not, as we have seen, have the power at T* to make the first conjunct of (10) true at T*. No one has such power. So it follows from (H) that S has the power at T* to make the second conjunct of (10) true at T*. But the second conjunct of (10) is identical with

(6) F (David is in Chicago at T) was true at every moment prior to T* .

So S has the power at T* to make (6) true at T*. Thus, if S has the power at T* to make (5) true at T*, then S has the power at T* to make (6) true at T*. Hence, (7) is true. And anyone who concedes that the antecedent of (7) can be true must also admit that S can have the power at T* to bring it about that the future-tense proposition F (David is in Chicago at T) was true at every moment before T*.

Notice that this argument depends only on the Ockhamistic theses (E) and (J) and on the principles (G), (H) and (K). Moreover, while (H) and (K) hardly require an argument, I have given what I take to be solid reasons for favoring (G) over its competitor (F) . So at the very least I have shown that it is reasonable for an Ockahamist to hold that we have power over the past, i.e., over certain temporally contingent past-tense propositions. And in the absence of good independent arguments against (G) , (H) or (K), I am prepared to say that my argument makes it epistemically obligatory for Ockhamists to accept (7) and the innumerable propositions that could be substituted for it.

Of course, the power over the past which I have argued for is rather limited, since an agent can have the power at t to make a given past-tense proposition true (or false) only if that proposition is neither necessary per accidens nor impossible per accidens at t. But Ockhamists all agree that there are many past-tense propositions that are contingent in this sense. At the risk of glossing over the differences which separate Ockhamists from one another, we can say simply that a past-tense proposition is contingent in the sense in question if its present-tense /65/ counterpart has not yet been true. (In what follows I will elaborate a bit on this.) On the other hand, the philosophers alluded to at the very beginning of this paper are far more liberal than the average Ockhamist in their claims about such contingency. They might insist, for example, that it is at least conceivable that someone now be transported to Socrates' death scene and find himself in a position to prevent Socrates from drinking the hemlock. Such power over the past obviously goes far beyond anything I have claimed here, and in this sense my argument is less interesting than their arguments are. However, I hope that it is clear that my position is also much more plausible than theirs, since it does not depend upon further dubious metaphysical presuppositions, e.g., that there can be two independent time dimensions, or that a person is literally a mereological sum of temporal parts, two of which might confront each other in a time travel scenario, etc. Instead, each of the assumptions I have employed seems to have substantial intuitive weight.

To summarize, then, anyone who accepts the Ockhamistic response to logical determinism can reject (7) only at the cost of having to give up (G), (H) or (K) . This is, at best, an uncomfortable choice.

I will now consider two fairly obvious objections to what has been said above:

(a) "Your view entails that contradictory propositions can be true at the same time. For suppose that David will not be in Chicago at T, even though he now (at T*) has the power to make it true that he will be in Chicago at T. In that case, the future-tense proposition NF(David is in Chicago at T) has been true at every moment before T* . But according to you, David now has the power to bring it about that F (David is in Chicago at T) was true at every moment before T*. Now suppose that David were to exercise this power. Then it would be the case that F (David is in Chicago at T) was true at every moment before T* . But, as we have just seen, it would also be the case that NF (David is in Chicago at T) was true at every moment before T* . It follows that contradictories will have been true at every past moment."

This conclusion does not follow. For just as p and possibly q can be true even though p and q is impossible, so too p and someone has the power to make q true can be true even though p and q is impossible. The fact that NF (David is in Chicago at T) has been true at every moment before T* entails only the no one will in fact make it the case that its negation was true at every moment before T* . It does not entail that no one has the power to do so. To say that David has the power at T* to make it the case that F (David is in Chicago at T) was true at every moment before T* implies only that there is a possible world w which shares the same history with our world at T* and is such that at T* in w David exercises this power. And, as I suggested above, the Ockhamistic position can be summed up in the claim that a careful explication of the phrase "shares the same history with our world at T*" reveals that it is plausible to suppose that there is such a world--even if David does not exercise the power in question at T* in our world. Of course, if he exercises this power at T* in w, then the /66/ proposition NF (David is in Chicago at T) is never true before T* in w. So my view does not entail that there is a moment--either in our world or in w--at which both of the contradictory propositions in question are true.

Nor does my view entail that David can alter the past, if we mean by this that he can bring it about that F (David is in Chicago at T) has always been true and that its negation has also always been true. That is, my view does not contravene (A*) above when it is construed according to its first reading. However, if we mean only that while NF (David is in Chicago at T) has in fact always been true before T*, its negation is such that David has the power to bring it about that it was always true before T*, then my view does entail that David has the power to alter the past. For this is just to say that PNF (David is in Chicago at T), while true at T*, is not necessary per accidens (i.e., part of our history) at T* . So my view entails, unsurprisingly, that (D), along with the second reading of (A*), is false. We might say that the Ockhamist holds that our past, but not our history, can be altered. Still, it is probably misleading to use the phrase "alter the past" in this sense, and so I am prepared to jettison it in favor of the more accurate locutions employed above.

(b) "Your view has the odd consequence that an agent can have the power to make a proposition true even though that proposition is already true. For suppose that David has the power at T* to bring it about that F (David is in Chicago) will be true at T. And suppose further that he exercises this power at T*, so that the proposition David is in Chicago is true when T is present. From this it follows that the proposition F (David is in Chicago at T) was always true before T* . Yet on your view David has the power at T* to "bring it about" or "make it true" that this proposition has always been true before T* . How can this be?"

Note, first, that my position is not the only one confronted by this difficulty. For instance, according to the "timeless" theory of truth every true proposition is eternally true and every false proposition is eternally false. So the "tenseless" proposition Jones is in class at T is, if true, true at every moment, including every moment before T. Yet one who holds such a view would presumably want to admit that Jones might have the power at T* to bring it about that Jones is in class at T is true, and hence eternally true.

Ockhamists, at least, have the resources to show that there really is no problem here. For, as I suggested above, they hold that the truth-value of any past-tense or future-tense proposition at a given time depends on the past or future truth-values of relevant present-tense propositions. For instance, the present truth-value of the proposition F (David is in Chicago at T) depends on whether the present-tense proposition David is in Chicago at T will be true at T. Moreover, the dependence in question is asymmetric. The present-tense propositions true at a given moment determine, according to this view, what is "presently" true at that moment, whereas the past- and future-tense propositions true at that moment do not. Instead, they depend on what was (will be) "presently" true at some past (future) moment or moments.

This notion of temporal dependence must, of course, be fleshed out more precisely, and I have attempted to do this elsewhere.16 But while the articulation of this notion is rather complicated, the intuition which underlies it is rather /67/ straightforward and attractive. Undergraduates, when first confronted with an argument for logical (or theological) determinism based on the necessity of the past, frequently respond that it is now true (or: God now knows) that David will be in Chicago at T because it will be the case at T that David is in Chicago. But the converse does not hold. That is, the following is thought to be clearly false: it will be true at T that David is in Chicago because it is now true (or: God now knows) that David will be in Chicago at T. Confusion is generated by the fact that these occurrences of the term "because" are often taken to signal causal dependence. But this construal does not do justice to the intuition in question, which has to do with logical (in a broad sense) rather than causal dependence.

Once we grant the validity of this intuition, we can then go on to say that at T* David brings it about that the proposition F (David is in Chicago at T) has always been true before T* by bringing it about that the proposition David is in Chicago will be true at T. And we can deny that David brings it about that David is in Chicago will be true at T by bringing it about that F (David is in Chicago at T) has always been true before T*. That is, anyone who brings it about that a future-tense proposition has always been true does so by bringing it about that an appropriate present-tense proposition is or will be true. So power over the past is not basic, but is rather parasitic on ordinary causal contribution to what occurs "presently." Of course, the proposition F (David is in Chicago at T) has always been true at the moment when David brings it about that it has always been true. But, as just noted, its past and present truth depend upon the future truth of the present-tense proposition David is in Chicago. Any possible world sharing the same history with ours at T* in which David is in Chicago is false at T is by that very fact a world in which F (David is in Chicago at T) is never true. So there is a perfectly straightforward sense in which David has the power at T* to bring it about that F (David is in Chicago at T) has always been true even though this proposition is already true. For its truth depends upon the future truth of the present-tense proposition David is in Chicago, and at T* David has the power to bring it about that this present-tense proposition will be true at T.

So my view may appear initially to have an odd consequence, but this appearance is illusory. What would be genuinely odd--and perhaps this is the underlying intuition on which objection (b) is based--is the claim that one could have the power to make a present-tense proposition p true at a time t when p would be true at t no matter what any agent were to do. This is not only odd, but impossible.

In summary, then, we have seen that a systematic framework for talking about the necessity of the past suggests a strong argument for logical determinism, and that one who espouses an Ockhamistic response to this argument can and should embrace the thesis that agents in fact have power--albeit limited power--over the past. Though someone might be tempted to use this result as an argument against the Ockhamistic position, I have tried to show in the final section that Ockhamists can satisfactorily handle at least the most obvious objections to the claim in question.17 /68/

1. See, e.g., Michael Scriven, "Randomness and the Causal Order," Analysis 17, 1957, 5-9; Michael Dummett, "Bringing About the Past," Philosophical Review 73, 1964, 338-359; Larry Dwyer, "Time-Travel and Changing the Past," Philosophical Studies 17, 1975, 341-350; and David Lewis, "The Paradoxes of Time-Travel," American Philosophical Quarterly 13, 1976, 145-152.

2. For example, William Rowe, in Philosophy of Religion: An Introduction (Encino, Calif., 1978), 154-169, and "On Divine Foreknowledge and Human Freedom: A Reply," Philosophical Studies 37, 1980, 429-430, puts into the mouth of the theological determinist a principle much like (A).

3. I am interpreting physical necessity and causal necessity in the following ways: p is physically necessary just in case it is a law of nature; and p is causally necessary at t just in case for some q (relevant causal conditions), q is true at t and it is physically, but not logically, necessary that if q is true, p is true.

4. Although I am primarily interested in unalterability here, it should also be noted for future reference that once a proposition becomes necessary per accidens, no one has the power to bring it about that it is or will be true, and once a proposition becomes impossible per accidens, no one has the power to bring it about that it is or will be false. So in this regard per accidens necessity and impossibility resemble logical necessity and impossibility. For a discussion of the role of accidental necessity in the formulation of an adequate analysis of omnipotence, see Thomas P. Flint and Alfred J. Freddoso, "Maximal Power," in Alfred J. Freddoso (ed.), The Existence and Nature of God (Notre Dame, forthcoming).

5. I will say that p entails q just in case it is logically impossible that there be a moment at which p is true and q is false. Similarly, p is logically equivalent to q just in case it is logically impossible that there be a moment at which p and q differ in truth-value.

6. See Aristotle, On Interpretation, chap. 11, and A. N. Prior, Past, Present and Future (Oxford, 1967), 128-134.

7. See Peter T. Geach, Providence and Evil (Cambridge, 1977), 40-66.

8. See my "Accidental Necessity and Logical Determinism."

9. Condition (b) simply ensures that there is a past at any moment at which an agent will be claimed to have power over the past.

10. The argument which follows would work just as well on the alternative assumption that an agent might have the power at T* to bring it about that (5) is or will be true at some t such that T > t > T* . But this assumption would needlessly complicate the argument, and so I have chosen to employ its simpler counterpart.

11. See Roderick M. Chisholm, Person and Object (Lasalle, I11., 1976), 65.

12. Person and Object, 84.

13. I say "genuine" here, since spurious counterexamples can easily be generated if (H) is given the following inaccurate reading: if S has the power to bring it about that p and q is true at t, then (i) S has the power to bring it about that p and not-q is true at t or (ii) S has the power to bring it about that q and not-p is true at t.

14. See "On Divine Foreknowledge and Human Freedom," Philosophical Studies 37, 1980, 289-296.

15. It is worth noting that (K) is incompatible with (F) above, since any proposition p is logically equivalent to its conjunction with a logically necessary truth. So, for instance, if (K) is true, then whoever has the power to make p true at t also has the power to make p and 2 + 2 = 4 true at t. But no one can have the power to make 2 + 2 = 4 true. So anyone who accepts (F) must reject (K) as well as (G). Given (K)'s intuitive appeal, this result provides us with another strong reason for rejecting (F).

16. See my "Accidental Necessity and Logical Determinism."

17. I wish to thank Thomas Flint, Richard Foley, Jorge Garcia, Philip Quinn and an anonymous referee for their helpful comments on earlier drafts of this paper.