 Jacques Maritain Center :
General Metaphysics /
by John Rickaby, S.J.
Jacques Maritain Center :
General Metaphysics /
by John Rickaby, S.J.
CHAPTER VI.
THE FINITE AND THE INFINITE IN BEING.
Synopsis.
(1) The true notion of the Finite and the Infinite.
(2) False doctrines about the Infinite. (a) That there is no such
thing, or at least no such notion. (b) That the notion is made up
empirically by the addition of finite quantities. (c) That the notion
can only be innate.
(3) Admittedly real difficulties connected with the notion of the
Infinite especially in regard to the so-called "sum of all
possibilities."
THERE is one more division of Being which we must discuss, before we
pass on to the second book of this treatise: it is the partition of
Being into Infinite and Finite. Straightway some readers will be
inclined to limit our inquiry to the one aspect of space, as though
infinity in extension were the only meaning of the term; and,
therefore, we wish distinctly to forestall such misconception, because
otherwise it may do a great deal to prevent our words from being
rightly understood. We have already used "perfection" as in some way
synonymous with Being; and if we say that our present investigation
concerns the difference between infinite and finite perfection in
things, we shall be conveying a much truer notion than if we spoke as
thougb
endless space and limited space were the two special objects of our
interest.
(1) The amount of discussion that has gone on in the world about the
finite and the infinite has been appalling, not simply on account of
its vastness, but more still because of the bewildering nature of many
of the speculations into which philosophers have wandered. It will,
therefore, be well to open the discussion by as concise an account of
the two ideas as possible, in order that the reader, having distinctly
before his mind what he ought to mean by the finite and the infinite,
may be enabled afterwards to take a few peeps into the wilderness of
confusion, with a steady confidence that it need not frighten him.
We began this treatise by making quite clear to ourselves the
signification and the reality of Being; also we have, in various
ways, been brought across the idea of negation or limit;
we need only put these two elements together, and we obtain the notion
of finite Being. That we ourselves are such Beings is brought
home most certainly to our consciousness by means of reflexion, no
matter how earnestly pantheists or monists may labour to persuade us of
our identification with the infinite.
The notion of Infinite Being is what we have next to make clear. The
success of the effort will not depend on the number of pages over which
we extend our account; if a few sentences amply suffice for our
purpose, all the better, except for the danger there is lest what is
contained within the compass of a single page should fail to secure the
attention
which it deserves, because it covers only one page. It would be
insulting to the reader to print in large capitals, or have fingers
drawn, pointing to the short passages that are important beyond the
measure of their length; but we may respectfully invite careful
advertence to the following paragraph which contains, substantially,
all the positive doctrine that General Metaphysics has to
deliver about the Infinite. In Natural Theology the subject has
to be further developed with painful elaboration.
Before, by denying more than a certain degree of perfection to Being we
got finite Being; and now if we deny our previous denial, and assert
unlimitedness of Being, we have got the idea of the Infinite, provided
we can satisfy one peremptory condition. We must give guarantee that
our new phrase is not self-contradictory; and this we cannot do by a
simple inspection and comparison of the two terms, "unlimited" and
"Being." Therefore we borrow from the treatise to which we have so
frequently to make recourse: we take from Natural Theology the
proposition that there actually exists an Infinite God, according to
inferences that are convincingly drawn. Thereupon, what otherwise would
have been no better than the suggestion of an idea, becomes a real
idea, and we are assured that our conception of "unlimited Being" is
valid. It is not a mere subterfuge like the pretence to pile finite
upon finite till the Infinite is reached: it commits us to no assertion
that the finite is made up of parts; it gives us simply Being which, as
such, is not confined within any bounds. While the idea so
formed really does attain to its object, we are free to confess that
it does so after a very imperfect mode, because it has to proceed by
way of negation, instead of positive intuition; and though the
negation, inasmuch as it is the negation of a negation, that is, of a
limit, is so far turned into something positive, yet for all that it
does not give us a direct positive conception of the infinite. Later on
we shall allow for all shortcomings, but here we are insisting upon the
success of our enterprise, so far as we have achieved a success. We may
now turn to the failures of others, which will take us more time to
consider, for error is often more roundabout than truth.
(2) (a) Some of the old pagan systems, even though they do not
explicitly deny the existence of the Infinite, implicitly deny it by
allowing only a finite quantity of material elements and certain
presiding spirits whose attributes declare them to be certainly finite.
Others argue that existence means determinate existence; that all
determination is limitation; and that, therefore, there can be nothing
actual which is not bounded.
It is, however, to the denial of our power to conceive the Infinite
that we may more profitably turn, because the arguments on this side
have about them a greater show of reason. Hobbes in his rough
leviathan-like way, quite ignoring the distinction between sensitive
and intellectual powers, thinks to crush, as with a sledge-hammer,
man's pretence to know the Infinite.{1} "Whatsoever we
imagine is finite. Therefore, there is no idea or
conception of anything we call infinite. No man can have in his
mind an image of infinite magnitude, nor conceive
infinite swiftness, infinite time, or infinite
force." The identification here of imagination, of the sensitive
picturing of infinite magnitude or velocity, with the intellectual
conception of the infinite is very characteristic. We all allow that we
cannot picture the Infinite; nor are we concerned to defend the
conceivableness of an infinite magnitude, nor yet of infinity in any
material order. Hence we quite disagree with the principles of a man
who, as Hobbes does, acknowledges no other actuality but what he calls
"body." He continues, "When we say anything is infinite, we signify
only that we are not able to conceive the ends or bounds of the things
named; having no conception of the thing, but of our inability. And,
therefore, the name of God is used, not to make us conceive Him, for He
is incomprehensible; but that we may honour Him. Also because
whatsoever we conceive has been perceived first by sense, a man can
have no thought representing anything not subject to sense. No man,
therefore, can conceive anything, but he must conceive it in some place
and endowed with some determinate magnitude." All contrary declarations
"are absurd speeches, taken upon credit, without any signification at
all, from deceived philosophers and deceived or deceiving schoolmen."
While differing widely from Hobbes as to the power of intellect above
sense, Hamilton agrees with him that we cannot conecive the Infinite.
For, he contends, "to conceive is to condition;" hence to think the
Infinite would be to condition the unconditioned, or to destroy it.
Nevertheless, we are bound to believe the Infinite, "believing
what we cannot prove," for "we have but faith, we cannot
know."{2} Our attempt to conceive the Infinite reveals a mere
impotence," "the negation of a concept," "a fasciculus of negations."
With these assertions Hamilton would have to reconcile what he says in
his Logic: "The manifestation of belief necessarily involves
knowledge; for we cannot believe without some consciousness or
knowledge of the belief, and consequently some consciousness or
knowledge of the object of belief."{3} This is rational, but it
warns us off the statement that simply we cannot know the
Infinite. Hamilton, however, is here pledged to a principle, which
occupies a great place in his system, and which he could not forego
without a notable retreat from a position long stoutly maintained.{4}
"The sum of what I have stated," he says, "is that the conditioned is
that which alone is conceivable or cogitable; the unconditioned is that
which is inconceivable or incogitable. The conditioned or the thinkable
lies between two extremes or poles, and these extremes or poles are
each of them unconditioned, each of them inconceivable, each of them
exclusive or contradictory of the other. Of these two repugnant
opposites the one is that of unconditional or absolute limitation, the
other that of unconditional or infinite illimitation." For example,
neither can we conceive a finite object which is an absolute whole or
an absolute part, nor can we conceive an infinite object, "for this
could only be done by an infinite synthesis in thought of finite
wholes, which would itself require an infinite time for its
accomplishment."{5} Here precisely we catch Hamilton tripping; for
addition of finites is not the only mode that man has of attempting the
idea of the Infinite, since we have already given another and a valid
mode. And this is the sufficient refutation of Hamilton, whose appeal
to Aristotle's{6} "The Infinite is unknowable as Infinite;" "The
Infinite is the object neither of the reasoning nor of
the perceptive faculty," will not avail him against the fact that
mankind have actually hit upon a means of conceiving the Infinite,
which manifestly does attain to the Infinite itself -- to the whole
Infinite, though not to a comprehensive, exhaustive knowledge of its
nature. No parts of it are left out, for it has no parts; still the
conception is partial in the sense that while it seizes the whole
object it does not wholly comprehend its nature.
In behalf of Hamilton, the defence which his pupil Mansel has to make
ought fairly to be heard, but it cannot be admitted to satisfy all
requirements. He contends{7} that Mill's attack is beside the mark, for
his great objection is, that Hamilton, instead of addressing himself to
the consideration of the concrete thing which is supposed to be
Absolute or Infinite, tries to prove "the unmeaning abstractions to be
unknowable;" whereas the truth is that "Hamilton maintains the terms
Absolute and Infinite to be perfectly intelligible as abstractions, as
much so as Relative and Finite, but denies that a concrete thing can
possibly be conceived as absolute or infinite." The abstractions are
knowable, "in the only sense in which abstractions can be known, by
understanding the meaning of their names;" but this meaning cannot be
intelligently applied by man to a concrete example, because "in order
to conceive the unconditioned existing as a thing, we must conceive it
as existing out of relation to every thing else, as one, simple, and
universal." The apology cannot be accepted, for the word Absolute
certainly can be applied to an object without excluding from it all
relation; the thing may be absolute under one aspect, relative under
another. This is clear enough in regard to finite natures: and with
respect to God, if we pass over the Trinity as belonging to Revelation,
all should admit that the Creator enters into what are conceived as
relations to His creatures; and if many theologians refuse to call
these relations real, it is only to save the appearance of
asserting any intrinsic change within the immutable God, or any real
dependency. Others, with the proviso, that the Divine attributes are to
be kept inviolate, say that the relation may be called real, in order
to signify that creation on the part of God is most really His work,
though He does not work after our way of passing from potency to act
and of depending on materials.
As a further instance of the view, that man can form no intelligible
notion of the Infinite, we may appeal to sermon literature, where we
shall find Kingsley addressing these words to a Christian public:{8}
"It is said God is infinite and absolute, and how can the finite
comprehend the Infinite? These are fine words, but I do not care to
understand them. I do not deny that God is infinite or absolute, though
what that means I do not know. But I find nothing about His being
infinite and absolute in the Bible. I find there that He is righteous,
just, loving, merciful, and forgiving: and that He is angry, too, and
that His wrath is a consuming fire; and I know well enough what these words mean." It is
not the way of Scripture to use philosophic terms to express a
doctrine; but there are plenty of texts setting forth the
illimitableness of the Divine Being, and these are taken by the early
Christian Fathers to mean that God is infinite, so that literally "of
His greatness there is no end."{9}
From Hamilton's doctrine that we must believe God infinite, though we
can form no conception of the Infinite God, onwards to Kingsley's
opinion, that a word which for us is empty of meaning need not be held
to declare a Divine attribute, the step is very easy. Dr. Martineau{10}
goes further still: his view is that instad of creation out of nothing
we must assume a sort of chaotic matter, coeval with God; and his
answer to Spencer's argument about the unknowableness of the Absolute
is, that it is enough to know God in His relation to His creatures.
"True, God, so regarded, will not in the rigorous, metaphysical sense,
be absolutely infinite. But we know no reason why He should be: and
must leave it to the schoolmen who worship such abstractions, to go
into mourning at the discovery." For Catholics, however, the Vatican
Council has inserted among its decrees a passage to the effect, that
God is a God of infinite perfection, the grounds for which doctrine may
be found in theological treatises, De Deo,{11}
while the meaning of the term "infinite" must be gathered, not from
Hamilton, or Kingsley, or Dr. Martineau, but from the philosophy which
the Church uses.
The Hamiltonian teaching about the inconceivability of the Infinite,
which has become widely diffused in this country, and which Professor
Huxley has lately described as having exercised a great influence
philosophically on his youthful mind, is akin to, but not identical
with, the Kantian distinction between the understanding, which judges
only according to the finite categories, and the reason which has
regulative ideas about the infinite, such, however, that we can
speculatively assert no real object corresponding to these subjective
ideas. Hegel, who kept the distinction between understanding and
reason, represented the true Infinite as not other than the finite, but
as that into which all finite objects are absorbed by the identifying
reason. He blames Kant for separating the infinite from the finite, and
making it a "transcendent," or an object beyond the reach of human
intelligence. Nevertheless, Kant's antinomies or contradictions have
largely prevailed: and they give Mr. Spencer, at the beginning of his
First Principles, his chief grounds for asserting the basis of things
to be the Unknowable.
(b) Next to the explicit rejection of a true notion, is its implicit
rejection by describing it in a way fatal to its essence: and such is a
description of the Infinite to which we have already referred, and
which makes it out to be the result of an indefinite
addition of finite quantities. This intellectual piling of Pelion upon
Ossa to some may seem a very sublime effort: but there is much truth in
Hegel's sarcasm against Locke on this point; that we must abandon the
occupation not because it is too sublime, but because it is too
tedious. Locke's teaching is{12} that "finite and infinite are looked
on as modifications of expansion," and that "as by the power we find in
ourselves of repeating as often as we will any idea of space, we get
the idea of immensity, so by being able to repeat the idea of any
length of duration, we come by the idea of eternity." Really this
process never brings us up to the notion of infinity: it leaves us at
some finite point, whence we look forward to a possible advance
indefinitely extending: but this is the indefinite, not the infinite.
At most it might be regarded as implying or presupposing the Infinite:
for, to take the example of space, if we assert that no matter how we
add space to space in our imagination, we can always go further in our
additions, we do in some sort insinuate that there is an unlimited
expanse to draw upon. If the idea does not involve self-contradiction,
about which there are grave doubts, then our way of conceiving infinite
space would be precisely by denying all limit to it. Locke, however,
omits this most necessary element in the process, and contents himself
with the indefinitely numerous parts. We are not, therefore, surprised
to find the patrons of this system equivalently admitting that they
have not got an idea of the Infinite, but only
a substitute for it; a fact which appears in Mr. Calderwood's polemic
against Hamilton. "It does not follow," he writes, "that since we have
not a clear and definite knowledge of the Infinite, we can have no
knowledge at all; we can have an indefinite knowledge of it; our notion
of the Infinite is a notion of that to which there is always something
beyond." To call the notion indefinite is to spoil it; only when a
previously determinate notion of the Infinite has been formed, can we
describe it as something such that, no matter what finite greatness we
assign to it, "there is always something beyond." This latter
description is a secondary account of the Infinite, and is insufficient
in itself, though, as we shall see presently, it has some authority in
Aristotle. Again, if we carry out the Lockian theory in Mr. Sheddon's
fashion,{13} and compare the acquisition of the idea of the Infinite to
climbing a mountain, which ever presents new peaks to our ascending
energies, then, with the same author, we must admit that "the Infinite
is for ever beyond our grasp." He " believes in its existence," but he
destroys the notion by which the object of the belief is expressed.
(e) To assert that the idea of the Infinite is innate in man, is about
the only resort left for those who, on the one hand, firmly hold that
he has the conception, and on the other, that his single way of
endeavouring to acquire it for himself by experience must be through
the addition of finite to finite. Descartes is a representative of the
a priori
theory of thinking: he maintains that only the Infinite Being could
have infused into our finite minds the knowledge of Infinity. From such
an inference we dissent; but otherwise what Descartes has to remark
upon the subject is not without some valuable hints. "As God alone," he
says,{14} "is the only true cause of all that is and can be, it is
clear that we shall be following the best course in our philosophy, if
from the knowledge of God Himself we try to deduce the account of the
things which He has created. Now that we may do so in security from all
danger, we must use the caution always to bear most carefully in mind,
that God is infinite and we altogether finite. Hence, if it should
happen that God reveal anything to us about His own nature, for
examples, the mysteries of the Blessed Trinity and of the Incarnation,
we shall not refuse to believe these truths which are beyond the reach
of our natural apprehension; nor shall we be in the slightest degree
surprised, that both in the immensity of His own nature, and in the
objects which He has created, there are many things which pass our
understanding. Never shall we weary out our minds in disputations about
the Infinite; for seeing that we ourselves are finite, it is absurd to
suppose that we can come to conclusions about it, and it would be
absurd in us to try to bound it within our comprehension. Therefore we
shall not be at pains to frame answers to those who ask whether, if a
line were infinite, the half of it would also be infinite; or whether
an infinite number be
odd or even; because it seems that no one ought to presume to have
ideas on these questions, unless he thinks his own mind to be infinite.
We for our part, in regard to all those objects to which, from some
aspect, we can discover no limit, shall not indeed call them infinite,
but shall look upon them as indefinite. For example, since we cannot
imagine an extension so great that it cannot be greater, we shall say
that things possible are indefinitely many." Upon this very point we
must presently enlarge a little, and the conclusion we shall try to
enforce is, that it seems safest to take refuge in the limitations of
our powers, and to confess our inability even to ask properly the
questions that are supposed to be so effective on one side of a
controversy or on another. We have to acknowledge not only insoluble
problems, but also problems that we cannot even state adequately.
However, before we take up this point, we have a few words to add. The
school of philosophers known as ontologists agree with the Cartesians
in teaching that we begin with the knowledge of the Infinite, and
thence descend to the knowledge of the finite; that our idea of the
Infinite is wholly a positive idea, and that the use of the negation
comes in when we conceive the finite as the negation of the Infinite.
The intuition of God and the infusion of ideas, which are the
postulates upon which the doctrines respectively rest, are both
contrary to sound psychological principle, or to speak more simply, to
the results of the most ordinary examination of experience. We must not
assume means which are beyond our powers, but must account for
each notion that we have, by assigning to it such an origin as we
discover in the workings of our own mind; and this we do when we trace
our idea of the Infinite to a conception of Being without limit. When,
however, we affirm that the notion of the finite comes before that of
the Infinite, lest we should seem to deny that correlatives can be
known apart, we must allow that a perfect perception of finite requires
us to observe that it is the opposite of the Infinite. Still there is a
less perfect knowledge of the finite to be had by observing the
difference in magnitude between two finite objects. To perceive that
one thing is smaller than another gives the idea of limitation; and
even though the idea of illimitation as applicable to Being, do not
then and there spring up, a sufficient contrast is at hand to produce
the notion of the finite. It may be only later that a deliberate effort
is made to give precision to the full contrary opposition of infinite
to finite; then an implicit idea becomes explicit.
(3) Without postulating any innate idea, we have shown how the Infinite
can really become an object of our knowledge; but at the same time,
because it was not an intuition, nor any fully comprehensive notion of
the object, that we proved to be ours, but only a sort of made-up idea,
needing the device of negativing all limit, it cannot surprise us, as
we have just heard Descartes remark, that our conception of the
Infinite leaves many puzzles to perplex the mind. We meet with no
downright contradiction of our doctrine: but we do meet with
difficulties apparently
beyond our powers of perfect solution. Unsolved difficulties, however,
cannot upset the partially acquired truths out of which they spring;
they show only the incompleteness of the knowledge, not that the
knowledge is not correct as far as it goes. The serious difficulties
about the Infinite do not so much begin with the One Infinite Being:
for He is declared to be without beginning or end, without parts or
composition, without change or any potentiality. But when we come to
the assertion of an infinite that has not this simple unity, but is
supposed to be constituted by finite parts, straightway strong
arguments appear against the validity of such a conception. St.
Thomas{15} indeed regards it as not demonstrable that creation could
not have been from eternity; but he distinctly says that there cannot
be an actually infinite number or multitude, and when he puts to
himself the explicit question, "Can the human intellect know an
infinity of objects?" he answers that "it cannot actually know an
infinity of objects, without numbering all the parts, and this is an
impossibility;" and that we can know Infinity in potentia only,
which is defined by Aristotle{16} to be "something such that those who
take any quantity of it have always more yet to take." This definition
is adopted by others, being rendered by Silvester Maurus{17} as
follows: "The infinite is that which always leaves something over and
above, so that he who subtracts from its quantity can always take more
and more, without ever exhausting the whole."
This quantitative infinite is quite a different thing from the
infinite perfection of simple Being -- simple not in the sense that it
is mere Being without determinate attributes that are mentally
distinguishable by us, while not really so, but simple in the sense of
uncompounded. Hence the Aristotelian definition really explains to us
no more than the indefinite; it tells us that no finite magnitude,
which we choose to name, will exhaust the possible extension of
quantity; but it does not tell us that there is an infinite extension,
nor even that infinite extension has a valid meaning. It informs us
only that, however far we stretch quantity, we can always stretch it
further. It gives us no more guarantee that we can predicate of it
infinite greatness, than that we can predicate of it, by reason of its
indefinite subdivisibility, infinite smallness, or parts infinitely
minute.
The inquiry has its direct bearing on the question of possibilities
which we treated in the last chapter. We are asked, Is their sum-total
infinite, or finite, or indefinite? If we reply finite, we seem to
limit the Divine power; if we reply indefinite, we seem to be using a
term that has reference only to human ignorance, and has no application
to the Divine knowledge; and therefore the remaining word, infinite, is
strongly urged upon our acceptance as the only one eligible. On the
threshold we may remark upon a frequent assumption which requires more
caution on the part of its friends than it generally receives. It is
taken for granted that there must be possible an infinite production as
the only
adequate term of omnipotence. But if this principle be urged
unqualifiedly, then omnipotence ought to be able to create another God;
and inasmuch as what is thus implied is the height of extravagance, we
have a right to affirm that God's power of creation has not an
absolutely illimitable term for its object. Here is a fact which at
least should be borne in mind while we are discussing the so-called sum
of all possibilities. Next we may premise, that from the point of view
of our limited capacities, "indefinitely many" forms a fair reply when
it is asked of us, How many things God can make? Never will so many be
assigned in numbers that He cannot produce more. We have the like
example of an indefinitely large multitude when we consider the
limitless subdivisibility, not perhaps of matter itself, but of
abstract, mathematical extension. In it there is no bound assignable by
us to the possibility of halving, and halving again, without ever
coming to a necessary stoppage. Once more, if it be asked, how many
thoughts will go through the mind of a person who is eternally to live
and to be mentally active, our powers of framing an answer at least
carry us as far as "indefinitely many."
But next, when we no longer consider our limited knowledge, which
easily allows of the indefinite, but God's knowledge which seems to
exclude indefinity, we feel driven to say that God could give a
definite reply to the query, "What is the sum of the possibles?" One
great advantage which He has over us certainly will enable Him to know
an infinite number or multitude, if that expression has an
intelligible meaning. For He does not number things successively: He
would not have to pass over successive steps in order to reach an
infinite number, if such a number have a real signification. To God,
then, perhaps, the sum of all the possibilities is infinite, or rather
infinitely infinite, in the sense that He contemplates an infinite
number of individuals in each of an infinite number of different
kinds.{18}
If by these considerations we could be driven into a plain
contradiction, it would be fatal to our philosophy: but if from them it
be proved only that about the infinite there are some questions which
we cannot satisfactorily, we will not say answer, but propose, then
that proves our knowledge to be restricted, but it does not discredit
the little that we do understand. If objectors cannot give a
sufficiently clear meaning to the inquiries which they are trying to
put to us, and by which they seek to reduce us to mental confusion,
then the limitation of their and our faculties may be betrayed; but our
theory about possibilities may still claim to be unshaken, so far as
ever we professed to have established a theory. It is enough,
therefore, if we succeed in showing that the almost flippantly made
interrogation, "What is the sum of all the possibilities on the supposition that their origin is in an infinite
God?" is dark, not with "excess of light," but with defect of light.
Perhaps it is absurd. At any rate, we shall content ourselves with
maintaining that its want of demonstrable intelligibility in any form
which we can give to it, is enough to bar its force as a decisive
objection to any doctrine. Others may meet the objection directly, but
we shall not attempt more than an indirect defence of our position
against the attack.
Recalling, as a thing to be kept in mind, our remark that omnipotence
cannot produce another God, we fasten upon the phrase, "sum of all the
possibilities," and demand proof that it is not incoherent in its
terms. We do not positively affirm that it is incoherent: but we ask to
have grave suspicions allayed which are against its coherency.{19} At
least it is an obscure combination of words, and we want more
clearness. It seems to ask what is the summation of a series which can
never end, and which cannot be submitted to any mathematical formula,
and which very likely would not gain much if it could be so submitted,
because mathematicians proceed by a convention in regard to the
infinite, leaving it to philosophers to explain the convention if they
can. The mathematician, as such, is quite
content to write 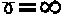 , and sometimes
, and sometimes 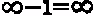 , and despises ultimate
explanation, because from his hypothetical point of view there
is no need of considerations that are in the present case
absolute . The theory of infinitesimals, or of absolute
ultimates in smallness, and the theory of limits -- either of these two
can be worked by the mathematician, who can work also with what he
recognizes to be, and calls, surds. He can allow the symbols for
impossible operations to enter into his workings and he ordinarily
considers it no necessary part of his business to venture any
philosophy about the deeper meaning of
, and despises ultimate
explanation, because from his hypothetical point of view there
is no need of considerations that are in the present case
absolute . The theory of infinitesimals, or of absolute
ultimates in smallness, and the theory of limits -- either of these two
can be worked by the mathematician, who can work also with what he
recognizes to be, and calls, surds. He can allow the symbols for
impossible operations to enter into his workings and he ordinarily
considers it no necessary part of his business to venture any
philosophy about the deeper meaning of 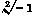 . For him the important point
about the infinite is that no finite quantity shall ever be allowed to
satisfy its requirements; and, as every one must see, this stipulation
is quite consistent with the impossibility of an infinite number, for
it exacts no more than the exclusion of a definite limit being set to
number in a particular case. Number in this instance is not an infinite
source actually existing, which, because it is infinite, enables us to
draw upon it indefinitely; it is only a magnitude capable of indefinite
expansion, but it is the expansion which gives the magnitude and
defines its limits at each stage: we are at liberty to push these
stages further and further, but it is a convention when the
mathematician supposes them infinitely advanced. We should need a more
philosophic explanation of that convention than the bulk of
mathematicians care even to attempt, before we could accept their use
of the terms as proof that a number literally infinite involves no
contradiction, or is not like a
surd. Mathematicians, then, at least leave us unsatisfied; professedly
many of them ignore the philosophic difficulties underlying their
convention. Suppose, therefore, we try for ourselves to discover what
is the meaning of all the possibles. We find that it is often treated
as the exhaustive term of inexhaustible power; the summation of an
unsummable series, or better perhaps, the last number in an arithmetic
progression, which ascends always by an increment of one, and has no
last term; the gathering up of all into one collection in spite of the
agreement that outside any assignable collection of the individuals,
there should always be more left to gather. Word our account of "all
the possibles" as we like, when we suppose them gathered into one sum,
the cautious mind will be slow to set aside its suspicions about the
validity of the expression. However, its defenders rest the case on
another consideration. They allow their inability to explain infinite
number; they appeal to the parallel instance of infinite Being, which,
nevertheless, we admit to be actually existent, though we cannot
comprehend it. Against such a subterfuge we have two things to say.
First, there are proofs producible for the infinite perfection of God;
but as God cannot create another God, there is a want of directness
about the argument from His own infinity to show that He can create, or
must regard as possible, an infinity of different kinds, or of
individuals under any one kind. Second, an infinity of finite objects
has difficulties which are avoided in the case of God who is one,
indivisible, uncompounded, and perfectly
simple in His essence. Hence with Him there is no constituting the
infinite out of parts: whereas the supposed infinity of possibles is
the result of an aggregation, which gives rise to endless and hopeless
perplexities, when inquiries are made about the results of adding or
subtracting units. The infinite number would have to be made up of
units, and these are elements which have furnished such difficulties
against the number itself that it seems safe to say, they have been
satisfactorily answered by no one; all attempt at reply rests on an
assumption which cannot rationally be justified. De Morgan is right in
his explanation of the numerals: they start, as he affirms, from one,
and then proceed by the addition of a unit at each successive advance.
Thus,
. For him the important point
about the infinite is that no finite quantity shall ever be allowed to
satisfy its requirements; and, as every one must see, this stipulation
is quite consistent with the impossibility of an infinite number, for
it exacts no more than the exclusion of a definite limit being set to
number in a particular case. Number in this instance is not an infinite
source actually existing, which, because it is infinite, enables us to
draw upon it indefinitely; it is only a magnitude capable of indefinite
expansion, but it is the expansion which gives the magnitude and
defines its limits at each stage: we are at liberty to push these
stages further and further, but it is a convention when the
mathematician supposes them infinitely advanced. We should need a more
philosophic explanation of that convention than the bulk of
mathematicians care even to attempt, before we could accept their use
of the terms as proof that a number literally infinite involves no
contradiction, or is not like a
surd. Mathematicians, then, at least leave us unsatisfied; professedly
many of them ignore the philosophic difficulties underlying their
convention. Suppose, therefore, we try for ourselves to discover what
is the meaning of all the possibles. We find that it is often treated
as the exhaustive term of inexhaustible power; the summation of an
unsummable series, or better perhaps, the last number in an arithmetic
progression, which ascends always by an increment of one, and has no
last term; the gathering up of all into one collection in spite of the
agreement that outside any assignable collection of the individuals,
there should always be more left to gather. Word our account of "all
the possibles" as we like, when we suppose them gathered into one sum,
the cautious mind will be slow to set aside its suspicions about the
validity of the expression. However, its defenders rest the case on
another consideration. They allow their inability to explain infinite
number; they appeal to the parallel instance of infinite Being, which,
nevertheless, we admit to be actually existent, though we cannot
comprehend it. Against such a subterfuge we have two things to say.
First, there are proofs producible for the infinite perfection of God;
but as God cannot create another God, there is a want of directness
about the argument from His own infinity to show that He can create, or
must regard as possible, an infinity of different kinds, or of
individuals under any one kind. Second, an infinity of finite objects
has difficulties which are avoided in the case of God who is one,
indivisible, uncompounded, and perfectly
simple in His essence. Hence with Him there is no constituting the
infinite out of parts: whereas the supposed infinity of possibles is
the result of an aggregation, which gives rise to endless and hopeless
perplexities, when inquiries are made about the results of adding or
subtracting units. The infinite number would have to be made up of
units, and these are elements which have furnished such difficulties
against the number itself that it seems safe to say, they have been
satisfactorily answered by no one; all attempt at reply rests on an
assumption which cannot rationally be justified. De Morgan is right in
his explanation of the numerals: they start, as he affirms, from one,
and then proceed by the addition of a unit at each successive advance.
Thus,
2 is the conventional sign for 1 + 1.
3 is the
conventional sign for 2 + 1.
4 is the conventional sign for 3 + 1.
Hence we can never get rid of the difficulties arising from the fact
that any number whatsoever is made up of separate units; and these
difficulties are serious.
The first Roman numerals are undisguisedly I, II, III.
Here it may be worth while to point out a defect in the expression,
that the numerals "tend to infinity." If we say that the asymptote
tends to touch its curve, or that a polygon of ever-multiplying sides
tends to a circle, the contact with the hyperbola and the contact with
the circle are in themselves terms which are most clearly intelligible
whatever may be
said of their being reached by the asymptote and the polygon. But if
any one says that ever-increasing number "tends to infinity," the
ultimate term itself here lacks the clear intelligibility which we
admitted in the former cases. It seems rather that the very law of
number,{20} or if some people prefer the word, of
multitude, should he that there shall be no term to which it can
tend as to its completion; just as it seems the law of the production
of parallel lines, that they should always as rigorously preserve their
distance, as if an inflexible bar held them apart. They have no law of
nearer and nearer approach, such as is apparent in the case of the
asymptote.
The force of the above arguments will be missed if the reader forgets
that they are purely sceptical, not dogmatic proofs against the
possibility of infinite multitude. They insist only on the two facts --
that there are unsolved difficulties against such infinity, and that we
cannot be compelled to sink these difficulties, because of some proof
aliunde, that there must be an infinite number or multitude. If
certain mathematical results seem to be against the latter assertion,
we plead in explanation, that these results depend on a priori
conventions which, at the time they were made, were not philosophically
analysed. The results follow deductively on the convention, but we wait
for the fuller analysis of that convention itself. As we have more than
once said, and must
repeat again because of the undue air of triumph with which the
consideration is pressed upon us, the difficulty of the infinite
multitude is not on the part of the Divine knowledge of it; if it be a
rational object of thought, God would know it all at once,
collectively, without successive summing up of parts. Still the parts
would be there and they are the obstacle; and we are quite unsatisfied
in mind when we are told to ignore the parts and regard only the whole,
as God would do. We are pertinacious in our assertions; the parts are
there, they make up the whole, and if their very nature appears to
throw grave doubt on the rationality of such a whole, to such doubts we
will cling until, we will not say our opponents, but our instructors,
make their instructions more intelligible to our powers of
understanding. For we cannot accept a proposition without some sort of
motive, intrinsic or extrinsic to the subject, and such as we can
understand.
Our position of non probatur, or not proven, against
those who hold an infinity of possibles may be further illustrated by
the failure of the attempt to translate eternity into clear terms of
time. If any one likes to say that eternity equals an infinity of
years, months, weeks, days, hours, or seconds, he has the power to
utter these words, but what do they signify? and what is their warrant?
and what is the excess of the infinity of years over the infinity of
seconds, sixty of which go to each minute? We should prefer to confess
that we do not know how to effect the translation of eternity into
time. Similarly we do not know which is the way to
express how God now looks comprehensively upon the thoughts of a
creature who is going to elicit thoughts throughout an eternity, that
is, who is going to posit a series which will never reach a final
limit, though it had a definite starting-point. Efforts to express
eternity in measures of time seem to lead us into fallacies comparable,
in part, to those whereby Zeno disproved the possibility of motion.
Motion continuous, successive, and without actually divided parts was
treated more or less like a fixed line, of co-existent parts, along
which it might be supposed to take place and have its resting-places.
But the fact is we cannot divide continuous motion itself into fixed
intervals of rest. Neither have we any right to speak of the
duration of an indivisible instant, nor to regard a finite
duration as made up of instants without duration, nor to make sundry
other suppositions which occasionally are made in dealing with those
very unique ideas, motion and duration, which are without first
part or last part, without co-existent parts, nay,
without any actual part at all. For motion of its own nature is
best conceived under the figure of an evenly-travelling point, which
leaves no record behind it, but simply goes ever uniformly forward. On
this subject we shall have to speak afterwards; at present we are only
calling attention to the fallacy of translating continuously successive
motion into co-existence and rest, and we are paralleling it with the
fallacy of translating the infinite into the finite.
Balmez has tried to illustrate the difficulties of an infinity of
finite parts. He says, in regard to
the assumed divisibility of finite space into infinite parts:{21}
"Absurdities if we suppose infinite divisibility, absurdities if we
suppose the opposite; obscurities if we admit unextended points,
obscurities if we deny them. Victorious in attack, reason is helpless
in defence, and unable to maintain an opinion. Nevertheless, reason
cannot be in conflict with itself: the proof of two contradictories
would be the absolute negation of reason. Therefore, the contradiction
is but apparent; but who shall untie for us the knot!"
We maintain that the foundation of the possibilities in an Infinite God
leads to no proved contradiction, but only to a question which is
suggested, yet seems incapable of clear formulation by the human mind.
And when we remember the mere artifice to which we must have recourse,
in order that we may have an idea of the infinite, which, while it
really attains its object, yet fails to comprehend its inmost nature,
we cannot be surprised that about this notion we have intimations of
questions to be put, but cannot clearly put them. If we may borrow a
rather distant analogy, we may use the illustration of a man who knows
sound only as it is heard, but who knows nothing about its mode of
propagation in a vibratory medium. He would ask some most unscientific
questions about the wonder that there should be a sound apiece for each
listener, about the disappearance of the sound as soon as it has been
heard, and about other matters equally vexatious to the educated man.
What seems a hopeless inquiry when sound is discussed with no approach
to an understanding of its physical conditions, becomes an intelligible
question only when an adequate hypothesis has been framed. So we may be
hopelessly muddling our brains over the infinite, because our
conception of its nature is so very indirect and inadequate. It does
not, then, seem extravagant to conclude, that perplexities concerning
the infinite and its relations to the finite, are no real
contradictions, but only attempts to think out problems that are beyond
our data. We are trying to stretch our terms till they reach to heights
and depths for which they are much too short. So when we are asked the
question with which we started this discussion, "What is the sum-total
of all the possibles?" we venture to maintain that the questioner has
no right to press that inquiry upon us, till he has satisfied us that
he has a determinate meaning to his words. Of course we must abide by
the law of excluded middle: and if the subject of the sentence
has a real meaning, and if in the case under discussion
non-finite is the same as infinite, then to the assertion, "The entire
number of possibles is either finite or infinite," we must yield our
assent. But we claim at present to take refuge in the conditionality of
the conditional particles, the two ifs: and we wait till these
can be replaced by the purely categorical statement, "The entire number
of possibles is either finite or infinite." We take refuge in Mill's
difficulty against the law of excluded middle, with this difference: he
supposes
his difficulty to be valid against the law itself, while we hold it to
be valid only against an uncertain application of the law. His
proposition is, that when a predicate is declared either to belong or
not to belong, to a certain subject, the assertion is open to the
exception, that possibly the term standing as subject is devoid of real
significance. This may be the defect of the phrase, "All the
possibilities."
It is the more needful to insist on the precise position which we take
up, for it borders so near on the Hegelian territory, that it may
easily be mistaken for one of its belongings. Hegel,{22} for example,
teaches us that "in the narrower sense dogmatism consists in the
tenacity which draws a hard and fast line between certain terms
supposed to be absolute, and others contrary to these. We may see this
clearly in the strict 'either -- or,' for instance, the world is either
finite or infinite; but one of these two it must be. The contrary of
this rigidity is the characteristic of all speculative truth. There no
such inadequate formulae are allowed, nor can they possibly exhaust it.
These formulae speculative truth holds in union as a totality, whereas
dogmatism invests them in their isolation with a title to truth and
fixity." Our way of dealing with the limitations of our understanding
is quite different from the Hegelian. Instead of postulating a power
higher than understanding, we simply do our best to make allowances
for its limitations, so that the partial truths we reach are considered
by us, not only as true, but also as partial, or as true only under the
recognition that they are partial. Where we recognize a distinct
contradiction between propositions, no matter how narrow, we refuse to
believe that this real contradiction can he overcome by a so-called
reason. Hence we cannot accept what follows on the passage just cited:
"The soul is the one just as much as the other, and in that way
neither finite only, nor infinite only: it is really neither one nor
the other." If finite and infinite are here referred to the same
aspect, and if the subject of the proposition, "soul," can be
taken, as "the sum-total of possibilities" cannot be taken, with
a perfectly clear and valid meaning; then on the principle of excluded
middle, the soul certainly is either infinite or not infinite,
"infinite" here meaning inferentially finite, for it cannot be
indefinite.
The only reason why we cannot apply the like dichotomy to "the sum of
the possibles," is because we cannot make sure about the meaning of
the phrase. Given that "the sum of the possibles" has a clear
signification and validity, then as we have said before, and now repeat
for the sake of emphasis, we should have to meet the difficulty from
the law of the excluded middle. Some, therefore, would allow the
infinity: others would say that non-infinite is not obviously the same
as finite, but may be the indefinite. We ourselves have not allowed the
question to go as far as this stage: we
have stopped the inquiry at its very birth by a demand for a perfectly
intelligible interpretation of the words, "the sum of the possibles."
On the ground above marked out we find a battlefield large enough for
the quarrels which probably philosophers will not settle till the end
of time, after which something higher than philosophy will enlighten
those who, during life, have been consistently something higher than
philosophers. Meantime we wait in humble acknowledgment of our
limitations.
{1} Leviathan, Bk. I. C. iii. p. 17.
{2} In Memoriam, Introductory Stanzas.
{3} Vol. IV. Lect, xxvii. p. 73.
{4} Metaphys. Lect. xxxviii. p. 373.
{5} Discussions, p. 53. "In our opinion the mind can conceive
and consequently can know, only the conditionally limited. The
unconditionally unlimited or the Infinite, and the
unconditionally limited, or the Absolute, cannot positively be
construed to the mind: they can be conceived only by thinking away
from, or abstraction of, those very conditions under which thought
itself is realized: consequently the notion of the unconditioned is
only negative -- the negative of the conceivable itself. For example,
we can positively conceive, neither an absolute whole, that is, a whole
so great that we cannot also conceive it as a relative part of a still
greater whole; nor an absolute part, that is, a part so small that we
cannot conceive it as a relative whole divisible into smaller parts."
In further making the Absolute to be the contradictory of the Infinite,
Hamilton only adds to the evidence that he is misconceiving the two:
"Absolutum means finished, perfected, completed: it thus
corresponds to the to holon and to teleion of Aristotle.
In this acceptation -- and it is that in which I myself exclusively use
it -- the Absolute is diametrically opposed to, is contradictory of,
the Infinite." Our doctrine is, that if we take the Absolute to be that
which is complete in its own nature, then the Absolute may be either
infinite, as in the case of the Divine nature, or finite, as in the
case of any created nature.
{6} to apeiron agnôston hê apeiron -- to apeiron
oute noêton oute aisthêton.
{7} The Philosophy of the Conditioned, pp. 110, 102, 103.
{8} The Gospel of the Pentateuch, Sermon II. Compare Dr.
Martineau's Study of Religion, Vol. I. pp. 400-416; Vol. II. p.
148.
{9} Psalm cxliv. 3.
{10} See the places lately referred to; also the Essays. Yet in
his own way, Dr. Martineau does teach that God is Infinite.
{11} Kleutgen gives the arguments in brief, De Deo, p. 186.
{12} Human Understanding, Bk. II. c. xvii.
{13} Three Philosophical Essays, Essay i. in initio.
{14} De Princip. Philosoph. Pt. I. n. 14.
{15} Sum. i. q. vii. a. iv.; i. q. lxxxvi. a. ii.
{16} Phys. iii. 6.
{17} Quaest. Philosoph. Lib II. q. xxxiii.
{18} "The right and duty to admit that something is and happens does
not depend on our ability, by combining acts of thought, to make it in
that fashion in which we should have to present it to ourselves as
being or happening. It is enough that the admission is not rendered
impossible by inner contradiction, and is rendered necessary by the
bidding of experience." Lotze, Metaphys. Bk. II c iii §
143.)
{19} "There is no enumeration of infinite numbers, and yet they are
not beyond the comprehension of God, whose intelligence is without
number." (St. Aug. De Civ. Dei, xii. i8.) "No species of number
is infinite, for in every case number is multitude measured by unity."
(St. Thomas, Sum. i. q. vii. a. iv.) The Conimbricenses take the
view that a quantitative infinity can be proved neither possible nor
impossible. (In Lib. VI. Phys. c. viii. q. ii.)
{20} Number is defined "multitude measured by unity." To escape this
definition some speak only of infinite multitude: but we have no belief
in trying to get over a real difficulty by a verbal distinction: it
would still remain to justify the assertion of an infinite "multitude."
{21} Fundamental Philosophy, Bk. III. c. xxiv,
{22} Mr. Wallace's Logic of Hegel, p. 56. Hegel pities Kant's
scrupulosity in limiting contradiction to reason and not referring them
to objects; he says the antinomies are real and are found in all
things.
<<
=======
>>
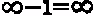 , and despises ultimate
explanation, because from his hypothetical point of view there
is no need of considerations that are in the present case
absolute . The theory of infinitesimals, or of absolute
ultimates in smallness, and the theory of limits -- either of these two
can be worked by the mathematician, who can work also with what he
recognizes to be, and calls, surds. He can allow the symbols for
impossible operations to enter into his workings and he ordinarily
considers it no necessary part of his business to venture any
philosophy about the deeper meaning of
, and despises ultimate
explanation, because from his hypothetical point of view there
is no need of considerations that are in the present case
absolute . The theory of infinitesimals, or of absolute
ultimates in smallness, and the theory of limits -- either of these two
can be worked by the mathematician, who can work also with what he
recognizes to be, and calls, surds. He can allow the symbols for
impossible operations to enter into his workings and he ordinarily
considers it no necessary part of his business to venture any
philosophy about the deeper meaning of  Jacques Maritain Center :
General Metaphysics /
by John Rickaby, S.J.
Jacques Maritain Center :
General Metaphysics /
by John Rickaby, S.J.