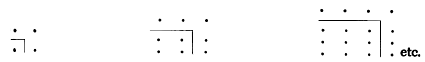
 Jacques Maritain Center :
A History of Western Philosophy Vol. I /
by Ralph McInerny
Jacques Maritain Center :
A History of Western Philosophy Vol. I /
by Ralph McInernyNow we turn to philosophers who, while not uninterested in the type of problem which bothered the Ionians, differ from them in striking ways. The Pythagoreans, for example, speak of the physical world as if it were constituted of numbers. Parmenides, on the other hand, overwhelmed by what he conceives to be logical truths, denies the reality of the world we see or think we see. His arguments against motion and multiplicity provide a difficulty for natural philosophy which is not solved until Aristotle. Moreover, his distinction -- between what appears to be and what is -- is destined to have a long history in Greek philosophy and beyond.
This group of philosophers takes its name from Pythagoras, a native of Samos in western Greece, but whose career was spent mainly in Italy. We speak of Pythagoreans rather than of Pythagoras, because little is known of the founder and, therefore it is difficult to assign to any individual the characteristic Pythagorean doctrines. The information we have from Aristotle about this school seldom begins with anything but the vaguest designation, e.g., "Certain Pythagoreans . . ." From an historical point of view, this presents difficulties. This is particularly true when we attempt to make use of Hellenstic testimonies, since the span of centuries, together with the anonymity of the members of the school, tends to blur the difference between very early Pythagorean teachings and later ones. For these were formulated with an eye to Plato, Aristotle and the Stoics.
The life of Pythagoras can be told rather briefly. Born in Samos, an island in the Aegean off the Ionian coast, where he is said to have lived until his fortieth year, perhaps 532/1 B.C., he then fled the tyranny of Polycrates. He then went to Croton in southern Italy where he was well received and, according to tradition, exercised no little political influence. His pupils there were said to have numbered some three hundred. The citizens of Croton finally revolted and set fire to a house in which the elder Pythagoreans were meeting, but Pythagoras himself escaped. He went to Metapontium where he died many years later.
The school that Pythagoras formed in Croton must not be thought of as the same sort as the "school" of Miletus. The Pythagorean community was structured more like life in a religious order. The community embraced men and women. There was a common doctrine but it was not to be divulged to outsiders; indeed, we have no mention of a Pythagorean writing anything until Philolaus at the end of the fifth century before our era. Pythagoras himself wrote nothing, but the practise of the society was to attribute every doctrine to its founder. Renowned for their secrecy, the Pythagoreans and a fortiori Pythagoras himself early became the object of mystery and speculation. Plato and Aristotle, consequently, are not in the habit of saying that Pythagoras said such-and-such, but that he is said to have said such-and-such, or, more usually, that the Pythagoreans or some Pythagoreans say such-and-such. There seems to be no doubt, however, that Pythagoras did live.
One very early testimony is that of Xenophanes who says of Pythagoras,
Once they say that he was passing by when a puppy was being whipped, and he took pity and said: 'Stop, do not beat it; for it is the soul of a friend that I recognized when I heard it giving tongue.' (Fr.7)
Thus, Pythagoras is said to have held the doctrine of the transmigration of souls; indeed, he is said to have remembered four previous incarnations of his own! Much later, Porphyry summarized his doctrine thus: (1) he believed in the immortality of the soul; (2) that it changes into other kinds of living things; (3) that events occur in definite cycles such that, to adapt the words of one commentator, the time will come when I will once more be writing these words and you will be reading them. That is, nihil novi sub sole: nothing is new and unique; (4) that all living things should be regarded as akin. There is a persistent tradition, beginning with Herodotus, that such doctrines were imported into Greece from abroad. Herodotus claimed that the doctrine of the transmigration of souls was borrowed from the Egyptians; but the Egyptians seem never to have held the belief themselves.
The belief that human souls could show up in other living things is connected with certain taboos or prohibitions observed by the Pythagoreans, such as abstention from meat and an injunction against associating with butchers. The testimony on these points in conflicting, however, since Pythagoras is said to have sacrificed an ox when he discovered the Pythagorean theorem. Later writers listed rules of conduct which were said to guide the Pythagorean community -- rules such as abstention from beans, and of smoothing out the impression left on one's bed, not wearing rings, not letting swallows nest under one's roof, etc. One that still has a peculiar force is this: "Speak not of Pythagorean matters without light."
We see, then, that the Pythagoreans were a community guided by a number of primitive and somewhat foolish rules of conduct, a secret society formed for the spiritual good of its members with a view to the survival of the soul. And this survival seems to involve reward or punishment for the deeds of one's present incarnation.
This is but half of the Pythagorean story, however; for to this mystic fervor was coupled an interest in science, particularly mathematics. Indeed, the society itself divided into two groups after the death of Pythagoras, the "Acousmatics" (hearers) and the "Mathematicians" (knowers). The former probably concentrated on the religious aspect of the society, while the latter devoted themselves to the more scientific aspect.
It is the peculiarity of the Pythagorean view of number which controls the scientific contributions of the society, and some at least of this view seems to go back to Pythagoras himself. Tradition has it that Pythagoras discovered that the chief musical intervals can be expressed in numerical ratios. If he arrived at this by measuring the length of the strings on a monochord, he would have expressed the octave as 2/1, the fifth as 3/2 and the fourth as 4/3. What we have to understand about Pythagoras' attitude is that he did not think of the numerical ratios as statements about sound by way of some application, but came to hold that there was an identity between number and sound. In other words, he did not arrive at any distinction between number and what is numbered, measure and the measured. This identification of things with numbers was to become the characteristic Pythagorean doctrine. The first four numbers, moreover, were thought to contain the whole nature of number, since 1 + 2 + 3 + 4 = 1O. When one gets to 10 he simply begins counting over again. Besides the Pythagorean theorem itself, Pythagoras seemingly can be credited with the discovery of the incommensurability of the diagonal and the side of the square. There is a story that one Hippasus of Metapontium was drowned for having revealed this to outsiders. Much speculation has been spent on why he should be so punished. One explanation is that the discovery of incommensurability of the alogon or irrational, was such a blow to the belief that there is a proportion or harmony in all things, that the initiates were particularly enjoined against revealing what could only seem a scandal.
The figure of Pythagoras is a shadowy one, not, as with the philosophers considered earlier, because of scanty information, but almost by design. He is the founder, the master, to whom all doctrines are attributed. (The Pythagoreans were famous for introducing statements with, "He himself said so.") And who is regarded as more than human, the son of Hermes. In one legend, for example, he is described as revealing his golden thigh. Soon the historical figure is lost behind the stories and our knowledge of what he taught is reduced to a view of the kinship of all things and an interest in mathematics which, apart from some mystical interpretations on the power of numbers, seems genuinely scientific. There seems to be as well the identification of things with numbers, leading perhaps to a belief in the harmony of all things -- a belief called into question by the discovery of the incommensurability of the diagonal and side of a square.
A remark attributed to Pythagoras describes perhaps for the first time an important aspect of what had been begun by Thales and was carried on by subsequent thinkers.
Life, he said, is like a festival; just as some come to the festival to compete, some to ply their trade, but the best people come as spectators, so in life the slavish men go hunting for fame or gain, the philosophers for the truth. (Diogenes Laertius, VIII,8){17}
We have here a distinction between the practical pursuits of men, the mark of which is activity and striving, and the pursuit of truth, described in terms of seeing or understanding for its own sake.
Because the philosopher wants to see, he must purge himself. The Pythagoreans held that as medicine purges the body, so does music purge the soul; and music -- proportioned sound -- is number. Number is the nature of all things. We must in this connection consider a lengthy passage from Aristotle.
Contemporaneously with these philosophers, and before them, the Pythagoreans, as they are called, devoted themselves to mathematics; they were the first to advance this study, and having been brought up in it they thought its principles were the principles of all things. Since of these principles numbers are by nature the first, and in numbers they seemed to see many resemblances to the things that exist and come into being -- more than in fire and earth and water (such and such a modification of numbers being justice, another being soul and reason, another being opportunity -- and similarly almost all other things being numerically expressible); since, again, they saw that the attributes and the ratios of the musical scales were expressible in numbers; since, then, all other things seemed in their whole nature to be modeled after numbers, and numbers seemed so be the first things in the whole of nature, they supposed the elements of numbers to be the elements of all things, and the whole heaven to be a musical scale and a number. (Metaphysics, I, 5)
Here Aristotle expresses what he learned of the Pythagoreans. They were interested in mathematics, Aristotle says, and this indicates an interest in the abstract, the formal, a science which does not have for its object the sensible things around us. Now the Pythagoreans, Aristotle says, thought of numbers as the stuff out of which things are made, as the Ionians had spoken of air and water as the primal matter out of which all things are fashioned. This is a difficult transition, and Aristotle gives us a few preliminary clues as to how it should be understood. Justice is a number, as is soul, and all other things; they are different arrangements of units and are thus made up of numbers. When Aristotle says that the Pythagoreans noticed that the attributes of the musical scale were expressible in numbers, he is speaking in terms of the recognition of a distinction which was most likely not known at the outset of the Pythagorean school. There is an unavoidable tension in the Aristotelian passage between the view that number is material cause and that it is somehow formal, applied to natural things, but itself different from natural things. Because they had not adequately distinguished between material and formal causes, the Pythagoreans seem to be making the same thing do service as both kinds of cause; number is that out of which things are made, and the particular arrangement of the elements is their nature. Consequently, we are faced with a doctrine according to which there is no distinction between natural science and mathematics, according to which the study of number tells us about the natural world as natural world. For the elements of number are the elements of all things.
What is meant by the elements of number?
Evidently, then, these thinkers also consider that number is the principle both as matter for things and as forming their modifications and their permanent states, and hold that the elements of number are the even and the odd, and of these the former is unlimited, and the latter limited; and the 1 proceeds from both of these (for it is both even and odd), and number from the 1; and the whole heaven, as has been said, is numbers. (986a15-21)
In this continuation of the previously quoted passage, Aristotle recognizes that number is matter and form for the Pythagoreans. What we must understand, if we are to grasp the identification of physics and mathematics, is the notion of oddness and evenness as the "elements of number," the relation between these and the number one and the numbers proper which follow from it.
If we must set aside the distinction between mathematics and physics to get an idea of Pythagoreanism, it seems that we must also abandon any sharp distinction between arithmetic and geometry. If we find this last shift considerably easier to accept, we must not think that the Pythagoreans anticipated any later view on the relationship between geometry and arithmetic. The Pythagoreans did not have, of course, anything like a simple system of notation for numbers. Very much later, Nicomachus indicates the difference between linear, plane and solid numbers in such a way that we understand that the source of this application of geometrical adjectives to numbers is quite pictorial. Linear numbers are obtained by setting down the unit once, twice, etc. Thus, 1 is a, 2 is aa, 3 is aaa, etc. Of course, the Greeks used letters of the alphabet as shorthand for such linear numbers (e.g., iota for 10; kappa for 20), but the linear numbers are basically what they stand for. Linear numbers are of one dimension and the unit is their principle. Plane numbers are generated from linear number and have three as their root. Here is Cornford's illustration of plane numbers.{18}
a a 1+2=3 |
a a a a a 3+3=6 |
a a a a a a a a a 6+4=10 |
a a a a a a a a a a a a a a 10+5=15... |
The first solid number is composed of four units and is illustrated by a tetrahedron. These remarks from later Greek mathematics give us an indication of the way in which the manner of depicting numbers leads to speaking of types of number progressing in terms of dimensions. The immediate relevance of these remarks is that they enable us to understand why the Pythagoreans spoke of odd and even numbers as square and oblong, respectively, and said that the former is finite, the latter infinite.
Further, the Pythagoreans identify the infinite with the even. For this, they say, when it is taken in and limited by the odd, provides things with the element of infinity. An indication of this is what happens with numbers. If the gnomons are placed round the one and without the one, in the one construction the figure that results is always different, in the other it is always the same. (Physics, 111,4,203a10)
If we start with the unit and enclose it with the first odd number, the figure obtained is a square; if we enclose the resultant figure with the next odd number, the figure remains the same, and the ratio of the sides is the same and the numbers obtained will be called squares, 4, 9, 16, and so on. Thus, square, odd and limit go together for the Pythagoreans. The following illustration will be of assistance here.
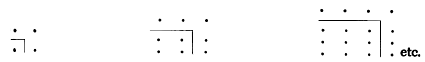
The oblong numbers, on the other hand, are obtained by beginning with 2 and enclosing it with the next even number. The continuation of this procedure is said to produce figures which are always different, that is, the ratio of the sides is never the same; the oblong numbers thus obtained are 6, 12, 20 etc.
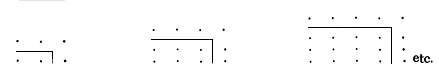
Thus, oblong, even and unlimited are grouped together by the Pythagoreans.
When we grasp that numbers are conceived in terms of different configurations of units in space, we can see how the Pythagoreans could have come to believe that the elements of number are the elements of all things. The crude way of making this identification was to take pebbles and form with them a picture of an object and, by counting the pebbles used, assign the number-nature of the object.
From Aristotle's account, we know that according to the Pythagoreans numbers have magnitude. "Now the Pythagoreans also believe in one kind of number -- the mathematical; only they say it is not separate but sensible substances are formed out of it. For they construct the whole universe out of numbers -- only not numbers consisting of abstract units; they suppose the units to have spatial magnitude . . ." (Metaphysics, XIII, 6, 1080b16) The illustrations we have seen indicate the identification of the arithmetical unit and the geometrical point; the point, however, while considered to be indivisible, is not without extension. Because of this, physical things could be looked on as in someway composed of such units as of their matter.
Before looking into the cosmology which followed from this view of mathematics, however, we must consider an important point made by Aristotle in his account of Pythagoreanism at the outset of his Metaphysics.
Other members of this same school say there are ten principles, which they arrange in two columns of cognates -- limit and unlimited, odd and even, one and many, right and left, male and female, resting and moving, straight and curved, light and darkness, good and bad, square and oblong. In this way Alcmaeon of Croton seems also to have conceived the matter, and either he got his view from them or they got it from him . . . For he says most human affairs go in pairs, meaning not definite contraries such as the Pythagoreans speak of, but any chance contrarieties, e.g., white and black, sweet and bitter, good and bad, great and small. He threw out indefinite suggestions about the other contrarieties, but the Pythagoreans declared both how many and which their contraries are. (Metaphysics, 1,5)
Alcmaeon of Croton, who is thought to have flourished at the beginning of the fifth century, was primarily concerned with medical matters, and one indication of his interest in contraries is to be found in his view that health is a balance of moist and dry, cold and hot, sweet and bitter, and so forth, and that sickness is the result of one contrary getting the upper hand. Certain Pythagoreans, as Aristotle says, were more systematic in pursuing the recognition of the role of opposites in the world and tried to summarize in ten oppositions the major types. We have already seen the reason for linking limit, odd and square, on the one hand, and unlimited, even and oblong, on the other. Even numbers are unlimited or infinite not, as Simplicius held, because the even number is infinitely divisible -- this is manifestly absurd -- but only in the sense that nothing prevents their being divisible into halves. The odd number is limited since, by adding one to an even number it prevents such equal division and, like three, the first odd number, has "a beginning, a middle and an end." Explanations of other contraries in the right or left column of opposites emerge when we look at Pythagorean cosmology.
If the elements of number are the elements of all things and odd and even are these elements, the number one, which is odd and even, must somehow be the source from which all things flow. Looked at from what for us would be the mathematical angle, we understand how bodies can be generated from points. The number one is represented by the point; in the order of linear numbers, 2 (..) would already be the line, where line is a cluster of at least two points. The first plane number, 3 (...) is the triangle; and 4, the first solid number, is the pyramid (...), The solid body, accordingly, is a number in the sense of a multitude of unit-points. Now, as has been mentioned earlier, just as the distinction between discrete and continuous quantity is not operative in Pythagorean mathematics, neither is there any distinction beween geometrical solids and physical bodies. Since their way of depicting numbers produced plane and solid figures, bodies and even physical bodies, were taken by the Pythagoreans to be composed of units and, consequently, these bodies are numbers. Aristotle is clear on this even as he objects to it. "For not thinking of number as capable of existing separately removes many of the impossible consequences; but that bodies should be composed of numbers, and that this should be mathematical number, is impossible. For it is not true to speak of indivisible spatial magnitudes; and however there might be magnitudes of this sort, units at least have not magnitude; and how can a magnitude be composed of indivisibles? But arithmetical number, at least, consists of abstract units, while these thinkers identify number with real things; at any rate they apply their propositions to bodies as if they consisted of those numbers." (Metaphysics, XIII, 8, 1083b10) Once more, the Pythagoreans did not consciously maintain that physical bodies are mathematical bodies, that the physically concrete is really the conceptually abstract -- they simply failed to make the distinction. The indivisibility of the unit-point is, as Aristotle pointed out, suspect if it is to possess magnitude. But this is what the Pythagoreans maintained. They were not consciously adopting a mathematical interpretation of the universe; for them mathematics was about the physical universe. The things in the universe are numbers and they are generated from the one. Pythagorean mathematics is a cosmology.
It is strange also to attribute generation to eternal things, or rather this is one of the things that are impossible. There need be no doubt whether the Pythagoreans attribute generation to them or not; for they obviously say that when the one has been constructed, whether out of planes or of surface or of seed or of elements which they cannot express, immediately the nearest part of the unlimited began to be drawn in and limited by the limit. (Metaphysics, XIV, 3, 1091a12)
Now this has in common with Ionian thought the fact that it is a way of explaining how the world began; "they are describing the making of a cosmos and mean what they say in a physical sense," as Aristotle adds. Aristotle is contrasting the Pythagorean view with the Platonic one according to which there are subsistent numbers, existing apart from physical bodies. Aristotle is under no illusions about the Pythagorean view of the extent of reality. Already in his account of their doctrine at the outset of the Metaphysics he wrote:
They employ less ordinary principles or elements than the physical philosophers, the reason being that they took them from non-sensible things (for the objects of mathematics, except those of astronomy, are without motion); yet all their discussions and investigations are concerned with Nature. They describe the generation of the Heaven, observing what takes place in its parts, their attributes and behavior, and they use up their causes and principles upon this task, which implies that they agree with the physicists that the real is just all that is perceptible and contained in what they call 'the Heaven.' (989b29ff)
We must keep in mind the identification made by Pythagoras himself of sounds and numerical ratios -- identification, not application -- lest we delude ourselves into thinking that the Pythagoreans have turned from the objects which concerned Ionian philosophy to other, more real entities. It is an appraisal of physical reality in both cases, not a conscious change of objects. The first stage of the cosmogonical process which Aristotle attributes to the Pythagoreans, consists of the formation of the first unit, though elsewhere he objects that the Pythagoreans are at a loss to describe the nature of his formation. (Metaphysics, XIII, 6, 1080b20) Subsequently, the unit draws in the unlimited and by imposing limits on it produces other units. As to the formation of the first unit, Aristotle mentions several possibilities: it could have been formed of planes or of surface, of seed or of some other elements. If composed of planes or surfaces, the first unit would be a solid. The supposition that the constituents of this first unit are seed would fit in with the location of "male" in the same column of opposites as limit. Its complement, the female, is the unlimited which it "draws in." The picture delineated becomes very much like the cosmogony of Anaximines when we learn that the unlimited is air and that the first unit breathes it in. Air or void is drawn in and keeps things apart, for it seems likely that the first unit grows and splits and is kept apart by the void or air. The continuation of this growth results in the universe we know.
Perhaps we have here the answer to the difficulty expressed by Aristotle: "For natural bodies are manifestly endowed with weight and lightness, but an assemblage of units can neither be composed to form a body nor possess weight." (De Caelo, III, 1) Kirk and Raven suggest that bodies would vary in weight according as they contained more or less void. This solution, of course, presupposes that the units have weight, and it is that assumption Aristotle is questioning. Aristotle is also perplexed by the fact that the Pythagoreans seem to leave qualitative distinctions unexplained.
To judge from what they assume and maintain, they speak no more of mathematical bodies than of perceptible; hence they have said nothing whatever about fire or earth or the other bodies of this sort, I suppose because they have nothing to say which applies peculiarly to perceptible things. (Metaphysics 1,8)
Even when we recognize that the Pythagoreans did not distinguish mathematical and physical bodies, their failure to explain sense qualities is a serious gap.
The Pythagorean view of the universe represents a significant shift from the geocentric view of Ionian philosophy. Fire, not earth, is the center of things and the earth is one of the stars for which night and day is caused by its circular motion around the central fire. They are said to have invented a planet, the so-called counter-earth, to bring the number of planets to ten, the perfect number. The counter-earth follows the earth in its path around the sun, always remaining invisible to us because of the bulk of the earth. "In all this," Aristotle comments, "they are not seeking for theories and causes to account for observed facts, but rather forcing their observations and trying to accomodate them to certain theories and opinions of their own." (De Caelo, II, 13) It is thought that the notion of the counter-earth dates from the time of Philolaus; another astronomical theory, that of the "harmony of the spheres" is considered to be of earlier origin in the school.
From all this it is clear that the theory that the movement of the stars produces a harmony, i.e., that the sounds they make are concordant, in spite of the grace and originality with which it has been stated, is nevertheless untrue. Some thinkers suppose that the motion of bodies of that size must produce a noise, since on our earth the motion of bodies far inferior in size and in speed of movement has that effect. Also, when the sun and the moon, they say, and all the stars, so great in number and in size, are moving with so rapid a motion, how should they not produce a sound immensely great? Starting from this argument and from the observation that their speed, as measured by their distances, are in the same ratios as musical concordances, they assert that the sound given forth by the circular movement of the stars is a harmony. (De Coelo, II, 9)
We do not hear this sound only because we have always heard it and are unable to contrast it with any opposed silence.
The Pythagoreans have lumped together unit and a point with magnitude, from which point the line is generated and so on to solids. Just as no differentiation is made between number and extension, so no distinction is recognized between mathematical and physical bodies, although this causes many rather obvious aspects of sensible bodies to go unexplained. The coming into being of our world is likened to the generation of the number series and the series of solids. The universe has grown from a primal unit which breathes in air or void and then splits up, imposing limits on the previously unlimited. The unit is considered to be male, the unlimited female. Earth is not at the center of the universe, but swings in a circular motion around a central fire, which motion produces day and night. In their movements, the heavenly bodies produce a wonderful music which has been singing in our ears since birth, and so is imperceptible by us.
In his dialogue, Parmenides, Plato gives us information which enables us to arrive at the approximate time of Parmenides' life. Plato says that Parmenides once visited Athens with his pupil, Zeno, when Parmenides was sixty-five and Zeno forty. Socrates was a young man at the time and had occasion to talk with the distinguished visitors; and that is the hook on which Plato hangs the dialogue. Since we know that Socrates was seventy when he was put to death in 399 B.C., the visit probably occurred about 451-449 B.C. With this information, we can arrive at the probable date of Parmenides' birth and say that he was in his prime about 475 B.C. This does not agree with the date assigned by Diogenes Laertius, but there is fairly general agreement that Plato can be relied on in this matter. The best argument for accepting the accuracy of Plato's information is that there would have been no need to have been so specific; and that since he was, it is more likely than not that the ages he gives are true.
Elea was a town in southern Italy, not far from Croton and Metapontium, where the Pythagoreans were influential. It is not surprising, therefore, to learn that Parmenides was a Pythagorean. It is said that Parmenides was converted to the contemplative life by Ameinias, a Pythagorean, although he is also said to have been a pupil of Xenophanes. Cornford (Plato and Parmenides, p. 28), speaks of Parmenides as "a dissident Pythagorean" and we will see the basis for this description in our analysis of Parmenides' poem. We have a good deal more to work with in the case of Parmenides than was true of any philosopher we have previously considered. We have the introductory portion of his poem which tells us in an allegorical fashion a good deal about what follows; moreover, large portions of the body of the poem have come down to us and we are able to arrange them in what seems to have been their original order. As we shall see, there is very little of the "poetic" in the Parmenidean doctrine; the prologue gives us a hint as to why he chose the form of presentation he did. As a poet, Parmenides is not held in very high esteem by knowledgeable scholars. Besides Xenophanes, there are only two philosophers who wrote in verse, Parmenides and Empedocles, who imitated Parmenides. Perhaps we can say of Parmenides what Aristotle said of his imitator: the poetry does not matter. We mention this to indicate that Parmenides presents no special instance of the problem raised in the first chapter because he expressed himself in verse. It is Simplicius, incidentally, to whom we are primarily indebted for our possession of so much of what Parmenides wrote; he introduced much of it in his commentaries on Aristotle. The prologue was preserved by Sextus Empiricus.
The fragment opens abruptly with the statement that Parmenides, the man who knows, is being borne in a car on the renowned way of the goddess through all the towns. Attended by maidens, Parmenides describes the sound made by the whirling axle and then, the daughters of the sun throw back the veils from their faces and leave the abode of night. At the entreaty of the maids, the gates of the ways of night and day are thrown open, Parmenides enters and is greeted by the goddess.
Welcome, O youth, that comest to my abode on the car that bears thee tended by immortal charioteers! It is no ill chance, but right and justice that has sent thee forth to travel on this way. Far indeed does it lie from the beaten track of men. Meet it is that thou shouldst learn all things, as well the unshaken heart of well-rounded truth, as the opinions of mortals in which is no true belief at all. Yet none the less thou shalt learn these things also -- how passing right through all things one should judge the things that seem to be.
The heightened tone of this prologue is thought to have been adopted in order to win respect for what is to follow. In fact, some think the prologue and the epic form were chosen to dress up an otherwise dull doctrine; but the prologue seems to be saying that Parmenides has in hand what must be regarded as a divine revelation. The goddess has spoken to him, even as a young man, and he is to learn all things. This is by way of conversion, and the opening sentence seems to suggest that Parmenides has sought in vain through many towns the knowledge that his heart desired, while all along, unbeknownst to him, he was being led on to the gates of night and day, and to the goddess whose revelation he would make known to us. The goddess instructs Parmenides in two ways: he is to know all things, both "well- rounded truth" and the opinions of men. The first is far from the usual thoughts of men; but it is the truth. The same cannot be said for the opinions of men. "But do thou restrain thy thought from this way of inquiry, nor let habit by its much experience force thee to cast upon this way a wandering eye or sounding ear or tongue; but learn by argument (logos) the much disputed proof uttered by me. There is only one way left that can be spoken of . . . ." We find in this passage, still part of the prologue apparently, an opposition between the two ways, that of opinion and of truth, expressed in terms of an opposition between the senses and argument or reason. The goddess asks that Parmenides eschew the senses and listen to the argument she will give in order that he might grasp the truth. As will appear, Parmenides is attacking the views of his predecessors, both those of the Ionian physicists and of the Pythagoreans whose cosmogony has many points of similarity with the Ionian.
The fragments which have come down to us can be divided according to the indications of the prologue into those pertaining to the way of truth and those belonging to the way of opinion. It has been a matter of much discussion as to why the poem of Parmenides should contain doctrine which he himself describes as utterly false. Why should he not confine himself to well-rounded truth and forget false opinions? Surely because the goddess revealed both; but why was this done? He is to learn all things, the false as well as the true; but above all, he is going to learn how to distinguish between the two. First we must consider the way of truth.
Come now, I will tell thee -- and do thou hearken to my saying and carry it away -- the only two ways of search that exist for thinking. The first, namely, that it is and cannot not be is the way of belief, for truth is its companion. The other namely that it is not and it must needs not be -- that, I tell thee, is a path that none can learn of at all. For thou canst not know what is not -- that is impossible -- nor utter it; for the same thing exists for thinking and for being.
We have here the initial statement on which all else ultimately depends for Parmenides; unfortunately, it is not a very clear statement. Let us take him to be saying that, if a thing is, it is and cannot not be, since it is impossible to think of something as not being.
Parmenides is convinced that it is nonsense to speak of something as not being, since it would seem that it somehow is and then is said not to be; but he will not allow that we can think of what is not as if it were. Indeed, if something can be spoken of and thought, it is, and that is all there is to it. "It needs must be that what can be spoken and thought is; for it is possible for it to be, and it is not possible for what is nothing to be. That is what I bid thee ponder." The goddess now suggests that the false way is twofold. The truth is that it is; the false that it is not, but there is a variation in falsehood insofar as men speak as if something could both be and not be.
I hold thee back from this first way of inquiry, and from this other also, upon which mortals knowing naught wander two-faced . . . undiscerning crowds who hold that to be and not to be are the same, yet not the same, and that of all things the path is backward-turning.
Cornford suggests that the attempt to reconcile being and non-being is actually an effort to accept both reason and the senses and that, once more, Parmenides is saying that we must leave the reports of the senses behind and rely on reason alone. Xenophanes and Heraclitus expressed some doubts about sense perception; Parmenides, however, goes far beyond them, for he maintains that nothing but falsity can be gotten from the senses. The senses give rise to the notion of opposites and contraries, to the belief that one thing is such-and-such and another is not such-and-such.
Thus far the doctrine of Parmenides seems utterly abstract and unrelated to the thought of his predecessors. He has insisted, in effect, on the difference between being and non-being. Being is being and non-being is non-being, and there is an end to it. Despite the differences, we can detect a continuity between Parmenides and his predecessors. The Ionians had each spoken of some one, primal thing whose modifications and states produce a multiplicity of things -- things which are contrary to one another: this one is not that one and vice versa. Nonetheless, there is some one nature, alive and divine, which pervades all things and which survives the ceaseless change of the many things perceived by the senses. When Xenophanes speaks of the one divine thing and the deceptiveness of the senses, he is not really going against the physical philosophers; he can be seen rather as laying even greater stress on the one which is stable and unchanging. Heraclitus finds unity in the proportionate and ordered changing of one opposite into another and back again. What is unchanging is change; and given the fact that one thing can change into another, opposites in a sense are not as other as we might think. The Pythagoreans, too, speak of the world as proceeding from a primitive unit, growing out of that unit in its multiplicity and variety. Parmenides reacted against all these views, though like his predecessors he begins with the one -- with what is. The one thing can be called being and the doctrine of Parmenides is that, when reason reflects on this one, it will seem that what philosophers had hitherto said is unacceptable to thought. The senses, of course, report multiplicity, the opposition of one thing to another and so forth; but this simply cannot be the case. Being is; non-being is not. Given the truth of that proposition, the falsity of earlier doctrines can be made manifest, even though the truth is surprising and far from the beaten path of men; for truth appeals to reason alone and can be attained only if we abandon the senses. Parmenides does not seem to be saying that anyone would explicitly maintain that nothing exists, that non-being is, but the variant of the position -- that being is somehow the same and yet not the same as non-being -- is held by many. As we shall see, it is this variant of the way of falsity which is at issue in the second part of the body of Parmenides' poem. His predecessors had all maintained in one way or another that being arose out of non-being.
What is the argument Parmenides is asked to heed?
One path only is left for us to speak of, namely, that It is. In this path are very many tokens that what is, is unborn and imperishable, for it is whole, immovable and without end. Nor was it ever, nor will it be; for now it is, all at once, a continuous one.
Being -- what is -- will be shown to have certain properties, namely, that it did not come to be nor will it cease to be. "For what kind of origin for it wilt thou look? In what way and from what source could it have drawn its increase? If being has come to be, we must be able to assign some source for it. "I shall not let thee say nor think that it came from what is not; for it can neither be thought nor uttered that anything is not." Can being come from nothing? This cannot be since we cannot say nor think that nothing is. We have already seen Parmenides maintain that what is thought and what is are one, so that non-being is unthinkable and unutterable. This may seem curious, since Parmenides insists that others have thought and said just this. Cornford suggests that what Parmenides means is that false statements -- statements about non-being -- have nothing to refer to and consequently are meaningless. Moreover, Parmenides adds, if being came from nothing, what prompted it to arise when it did? There is no reason for it to come forth at one time rather than another. Being, therefore, is simply being all at once and ever, or there is simply nothing and always will be.
Our judgment thereon depends on this: 'Is it or is it not?' Surely it is adjudged, as it needs must be, that we are to set aside the one way as unthinkable and nameless (for it is no true way), and that the other path is real and true. How then can what is be going to be in the future? Or how could it come into being? If it came into being, it is not; nor is it if it is going to be in the future. Thus is becoming extinguished and passing away is not to he heard of.
Being cannot come to be because there is nothing from which it could come save nothing and it cannot come from that. Being is, right now and always the same; tenses have no meaning in speaking of being since it has neither a past nor a future. There are other properties of the one being.
Nor is it divisible, since it is all alike, and there is no more of it in one place than in another, to hinder it from holding together, nor less of it, but everything is full of what is. Wherefore it is wholly continuous; for what is, is in contact with what is.
The Pythagoreans explained the coming into being of the many by saying that the primal unit breathed in the void which then served the function of keeping the split-up units separated from one another. Thus, in this view, there are interstices in being, and the void of non-being is employed to explain a multiplicity of things. Parmenides will have none of this. Being is a plenum indistinguishable into parts as if there were more being here than there or as if something intervened between what is and what is. Being is one, homogeneous, the same throughout, containing and permitting no void or non-being. We see once more that Parmenides does not want to go beyond the one with which his predecessors can be said to begin. First there was some one nature and then by one process or another, it broke up into many things and so forth. Parmenides wants to begin and end with the one being which is wholly the same, unique, admitting no gaps or distinctions within itself, utterly homogeneous.
Not only is being ingenerable and incorruptible, it does not move.
Moreover, it is immovable in the bonds of mighty chains, without beginning and without end; since coming into being and passing away have been driven afar, and true belief has cast them away. It is the same, and it rests in the selfsame place, abiding in itself. And thus it remaineth constant in its place; for hard necessity keeps it in the bonds of the limit that holds it fast on every side. Wherefore it is not permitted to what is to be infinite; for it is in need of nothing; while, if it were infinite, it would stand in need of everything.
Being cannot be undetermined, since then it would require a determination from something else. Rather it is limited and thus complete, something which leads Parmenides to provide us with an image of the being he is speaking of. Since then it has a furthest limit, it is complete on every side, like the mass of a rounded sphere, equally poised from the center in every direction; for it cannot be greater or smaller in one place than in another. For there is nothing that could keep it from reaching out equally, nor can aught that is be more here and less there than what is, since it is all inviolable.
The well-rounded truth -- that what is, is and cannot not be -- involves a view of being as a solid sphere in which there is an equal distribution of being throughout, no gaps, no imbalance. This is the truth of the matter, whatever the senses report. There is one being, unique, immobile, absolutely unchangeable and indivisible. These properties have been deduced from the original statement by showing that any other possibility is contradictory.
Thus Parmenides has rejected the dualism which defines the Pythagorean doctrine. Indeed, it can be seen that he has chosen one column and rejected the other. The left-hand column of opposites contains limit, unity and resting or immobility -- the very contents of the way of truth. Parmenides is, in the words of Aristotle, a nonnatural philosopher, since it is not the world of change which interests him, that world being for him an illusion from which we are freed by the way of truth. The being we come to know by reason is one, timeless, unchanging, devoid of all perceptible qualities, a motionless sphere of completely homogeneous mass.
With Parmenides, philosophy reaches a crisis that is acutely felt by all who follow on him. The validity of the senses has been called into question and with them, the world of opposites, of hot and cold, smooth and soft, and all other perceptible contraries. We are driven by cold reason to a view of being which is austere and uncompromising and which threatens the beginnings of natural science achieved by Parmenides' predecessors. We will see later the central place Aristotle accords to Parmenides and how important he feels is his own resolution of the Parmenidean dilemma which makes change and multiplicity impossible.
The methodology of the Parmenidean poem must be carefully considered. For the first time, we have a use of reasoning which moves from an initial statement to inescapable consequences. This dialectic is something which will be employed by Zeno, the pupil of Parmenides, and we must see in it the beginnings of what comes to be called logic. The basic procedure is a reduction to absurdity. Parmenides makes progress by showing that views contrary to his own involve impossible consequences; when we see this, we are prepared to accept as the truth the doctrine he would maintain: what is, is and cannot not be. What is not, is not and cannot be. There is no way in which being and non-being can be construed as in any way the same. To maintain that being is generable or corruptible, measured by time, divisible or mobile is to fly in the face of these premises. We must then accept the premises as true and abandon all hope of reconciling with them the world we see around us. Nonetheless, the poem of Pa~enides has things to say about the ordinary world. "Here shall I close my trustworthy speech and thought about the truth. Henceforward learn the beliefs of mortals, giving ear to the deceptive ordering of my words."
Before turning to the way of opinion, that part of his poem in which Parmenides sets forth a doctrine about the world around us, a doctrine revealed to him by the goddess so that no other mortal will appear wiser than Parmenides, there is an important question to be asked. Is the one being of which Parmenides speaks immaterial? In favor of an affirmative answer is the sharp dichotomy Parmenides draws between what the senses grasp and what is real for mind. The latter, the only true being, is then stripped of all sensible qualities and has none of the properties we should normally associate with the corporeal or material. On this interpretation, Parmenides' example of the sphere would be just that -- an example, a simile, an aid to understanding -- but not a literal description of the one being. Perhaps it is safer to hesitate here and ask ourselves whether Parmenides can seriously be taken to have arrived at the notion of a wholly immaterial being, the only being that is. True enough, in speaking of the one being, he denies of it many properties of sensible bodies, a process which may suggest all too easily to us an incorporeal, nonspatial reality. We may even want to suggest that Parmenides was groping towards such a notion, but the fragments we have do not permit any categorical statement to the effect that Parmenides has arrived at the recognition of immaterial reality. Aristotle was of the opinion that the Eleatics, Parmenides and Melissus, were aware of no reality beyond the corporeal although they were forced by their dialectic to the recognition of the need for something unchanging as a ground for true knowledge. (De Caelo, III, 1, 298b14) What they did was to question the validity of sensation, deny sensible qualities of the one being which nevertheless remained a body. In other words, the Parmenidean being retains the note of spatial extension and, if doubtless a strange one, is nevertheless a body. As with the Pythagoreans, Parmenides speaks in such a way that what he has to say could be taken to be applicable to non-physical being -- provided of course that there is such being and we have some cognitive access to it. This recognition, on our part, is no argument that the Pythagoreans themselves or Parmenides recognized there was such being. In short, Aristotle's opinion that they made no such explicit recognition seems deserving of acceptance. This is not to say that the procedure of the Pythagoreans and of Parmenides was not destined to have great influence on later attempts to achieve scientific knowledge of non-physical being. And, again, the method of Parmenides and his immediate follower, Zeno, had no little influence on the development of what came to be called logic.
In the way of opinion or of seeming, the poem of Parmenides passes from what is accessible to reason alone to what the senses report. The goddess has urged Parmenides to give ear to the deceptive ordering of her words, which may refer to the earlier remark that
language about what is not is meaningless and that, in this part of the poem, we find a doctrine concerning things which, truly speaking, are not. The reason given in the poem for this part of the revelation is that Parmenides must not appear less than anyone else. There has been much conjecture about the motive for the natural doctrine of Parmenides, and of course it will occur to one that Parmenides will enjoy a poor sort of superiority if he surpasses others in the order of falsehood. Burnet feels that Parmenides is here giving a review of popular beliefs concerning the physical world, that it is, in effect, a sketch of the Pythagorean cosmology. Kirk and Raven are unimpressed by this estimate, since they fail to find the characteristic notes of the Pythagorean doctrine. More positively, they point out that the ancients uniformly considered the cosmology to be of Parmenides' own devising. Aristotle feels that Parmenides, in the way of opinion, is attempting an explanation of the way being appears to man with his senses, something he could do without in any way changing his mind that this world involves features which are contradictory to pure reason. Whatever the explanation of this second part of the body of his poem, it does not contain the influential portion of Parmenides doctrine; indeed, neither Melissus nor Zeno seems to exhibit the slightest interest in anything save the content of the way of truth. Moreover, much less of the way of opinion has come down to us than of the way of truth. It is mainly for this reason that we shall content ourselves with having given some slight indication of the difficulties posed by the existence of the way of opinion, and not go into the many attempts to make sense out of the few fragments of it we possess. Whatever his motives for setting it down, Parmenides' physical doctrine seems to be pretty nearly the same sort of attempt as his predecessors had made. What is utterly distinctive of the Eleatic philosopher is the dilemma he poses for anyone who would take seriously what his senses tell him of the world. All that is false, Parmendies has argued; what is, is one, immobile, indivisible, atemporal; it has neither been generated nor can it ever be destroyed. To put it most succinctly, Parmenides has called into question the existence of change and multiplicity. Change is impossible, for if something has come into being, it must first of all not have been; that is, we would have to say that something came from nothing. Multiplicity too is unacceptable. If there are two things, two beings, how do they differ? They cannot differ in being, because that is what they have in common; they cannot differ in nothing, since that is no difference. There can be no difference in what is, accordingly, and we must recognize that being is a monolithically unique body. This type of doctrine and the argumentation which sustains it is the major contribution of Parmenides to the development of Greek philosophy. We can now turn to the defence and development that doctrine received in the hands of the followers of Parmenides.
We have seen that Zeno made a journey to Athens with Parmenides when the latter was sixty-five years old. At that time, Zeno was forty and, on the basis of the earlier analysis, we can place Zeno's birth at approximately 490-485 B.C. The story of his life, meager as it is, parallels the little we know of his master's. Zeno is a native of Elea, and a converted Pythagorean. In his Parmenides, Plato speaks of a book written by Zeno in which the pupil essays the defence of his master against those who object to his doctrine of the one, a defence that pays the attackers back in their own coin, for, as they had maintained that many absurdities follow from the position of Parmenides, so Zeno is intent to show that absurdities equally if not more great follow from adopting a view opposed to that of Parmenides. Tradition has it that Aristotle, in a lost dialogue of his, credits Zeno with being the founder of dialectic or logic. Zeno seems to have been primarily concerned with showing that impossible contradictions issue from our acceptance of the reality of motion and of multiplicity. Let us turn immediately to the consideration of some of these arguments which have come down to us.
As we have indicated, the arguments of Zeno are directed against multiplicity and motion. What is the multiplicity against which Zeno argues? There are at least two possibilities. We may take Zeno as arguing against the possibility of there being many things in the macrocosmic world around us; or, and this is the more likely one, Zeno is arguing against the Pythagorean doctrine that things are numbers and consequently aggregates of unit-points. Thus anything is a plurality of such monads which in themselves are indivisible but have position, that is, are in space. With this in mind, we can turn to a few of the more than forty arguments Zeno is said to have devised against multiplicity.
If there is a plurality, things will be both great and small; so great as to be infinite in size, so small as to have no size at all. If what is had no magnitude, it would not even be. For if it were added to something else that is, it would make it no larger; for being no size at all, it could not, on being added, cause any increase in size. But if it is, each thing must have a certain size and bulk, and one part of it must he a certain distance from another; and the same argument holds about the part in front of it -- it too will have some size and there will be something in front of it. And it is the same thing to say this once and to go on saying it indefinitely; for no such part of it will be the last, nor will one part ever be unrelated to another. So, if there is a plurality, things must be both small and great; so small as to have no size at all, so great as to be infinite.
Zeno may here be seen as putting his finger on the difficulties which attend the attempt to identify mathematical and physical bodies. Any determinate thing will be a given number of units; thus it will be finite in arithmetical and geometrical quantity, such-and-such a number, and a body of determinate size. Zeno wants to show that if we hold that the units have magnitude, things composed of them are going to have to be infinite in size. Between any two units there must be room for another, and so on to infinity. This point is made explicitly in another fragment.
If things are a many, they must be just as many as they are, and neither more nor less. Now, if they are as many as they are, they will be finite in number. If things are a many, they will be infinite in number; for there will always be other things between them, and others again between those. And so things are infinite in number.
In mathematical magnitude, we never run out of points; between any two points on a line, an infinity of points can be designated. When physical bodies are spoken of as composed of points having magnitude, we apply the geometrical doctrine and arrive at the need to say the physical body is infinite in size. If, on the other hand, we try to elude the difficulty by denying that the points of which physical bodies are composed have size, then we would be hard pressed to explain how something having size is made up of parts having no size. This view of things as pluralities of units, then, involves the idea that bodies are infinite in size and that they have no size at all.
On this interpretation, then, Zeno is looked on as defending the views of his master against the attacks of the Pythagoreans. The plurality which involves contradictory consequences is the plurality of units physical bodies are said to be. But Zeno was also concerned to defend his master's doctrine that being is immobile. His procedure is the same; the acceptance of motion involves one in contradictory consequences. Zeno is credited with four arguments against motion; they are preserved by Aristotle and are important enough in themselves and for subsequent philosophy to be examined in their entirety.
The impossibility of traversing a race track --
You cannot traverse an infinite number of points in a finite time. You must traverse the half of any given distance before you traverse the whole, and the half of that again before you can traverse it. This goes on to infinity, so that there is an infinite number of points in any given space, and you cannot touch an infinite number one by one in a finite time.
Once more, we have the consequences of the Pythagorean identification of the mathematical and physical drawn out. If a length is infinitely divisible and if a physical length is composed of points having magnitude, then the traversal of a finite space implies the traversal of infinite spaces. Aristotle, in first mentioning this paradox in Book Six of his Physics (233a21), sets it aside by pointing out that Zeno should have put the same question to time that he puts to space. The finite time in which one is held to traverse an infinite distance is itself infinite in much the same way as the finite distance is. That is, Zeno begins with some such situation as this. I run a mile in five minutes. The mile, of course, is a finite distance but it can be infinitely divided into lesser distances. Therefore, in five minutes, a finite time, I traverse an infinite distance. But, Aristotle observes, the finite time, five minutes, is divisible to infinity in much the same way that the mile is. This is not, of course, anything like a complete answer to the dilemma, nor did Aristotle think it was. In a neglected passage in Book Eight of the Physics he returns to the matter, pointing out the inadequacy of the previous reply. (263a4ff.)
Achilles and the tortoise --
Achilles will never overtake the tortoise. He must first reach the place from which the tortoise started. By that time the tortoise will have got some way ahead. Achilles must then make up that, and again the tortoise will be ahead. He is always coming nearer, but he never makes up to it.
This is not essentially different from the previous argument, only more complicated, since we have to do with two moving bodies and division of space in fractions other than halves. Once more, it is directed against the view that physical magnitude has the same properties as mathematical extension.
The arrow --
The arrow in flight is at rest. For, if anything is at rest when it occupies a space equal to itself, and what is in flight at any given moment always occupies a space equal to itself, it cannot move.
Once more, the assumption is that time is composed of moments in the way that the line has been taken to be composed of points.
The moving rows --
It will be best to approach this argument by way of a diagram.
(a) AAAA
(b) BBBB-->
(c) <-- CCCC
Imagine a race track on which the four bodies in (a) are stationary, and consider the bodies in rows (b) and (c) to be moving past them in opposite directions, with those of (b) occupying a position between the goal and middle point of the track and those of (a) between the midpoint and the start. With this example, Zeno wants to show that half a given time is equal to twice the same time. As the race ends, the first body in (b) reaches the last body in (c) at the same moment as the first in (c) reaches the last in (b), but at this moment, the first in (c) has passed all the bodies in (b) whereas the first in (b) has passed only half the bodies in (a). Thus, the first in (b) has taken up only half the time as the first in (c). The movement of (c) with respect to (b) is double the movement of (c) with respect to (a), since the first body of (c) passes all the bodies of (b) and half those of (a). Thus, while each body in (b) has passed two in (a), each body in (c) has passed four in (b). Zeno can be taken to have proved that it is impossible to think of space and time as composed of indivisible units. That is, he is making the point that the sensible and the mathematical are not the same thing, that the properties of the latter cannot be attributed to the former. It is just this confusion that the Pythagoreans seem guilty of, and Zeno is justified in drawing out the implications of that confusion. Here is the way Aristotle deals with this argument.
The fourth argument is that concerning the two rows of bodies, each row being composed of an equal number of bodies of equal size, passing each other on a race-course as they proceed with equal velocity in opposite directions, the one row originally occupying the space between the goal and the middle point of the course and the other that between the middle point and the starting-post. This, he thinks, involves the conclusion that half a given time is equal to double that time. The fallacy of the reasoning lies in the assumption that a body occupies an equal time in passing with equal velocity a body that is in motion and a body of equal size that is at rest; which is false. (Physics, VI, 9, 239b33)
Although Melissus moves us geographically back to the area where Greek philosophy began, his acceptance of the doctrine of Parmenides dictates his inclusion with the Italian school. He led the Samian fleet that defeated that of the Athenians in a battle fought in 441-440 B.C. We know little else of the life of this man; and it is dubious whether he was ever in personal contact with Parmenides, although he is said to have been his pupil. This is thought to have been based on what he wrote rather than on any knowledge of, or association with, the Eleatic philosopher.
We have already seen that Parmenides maintained that being was finite, meaning by this that it was perfect of itself and needed nothing outside itself in order to attain perfection. Melissus, many of whose fragments are simply repetitions of Parmenides, departs from his master on this matter of the finitude of being.
Since, then, it has not come into being, and since it is, was ever, and ever shall be, it has no beginning or end, but is without limit. For, if it had come into being, it would have had a beginning (for it would have begun to come into being at some time or other) and an end (for it would have ceased to come into being at some time or other); but, if it neither began nor ended, and ever was and ever shall be, it has no beginning or end; for it is not possible for anything to be over unless it all exists. (Fr. 2)
This argument did little to earn for Melissus the esteem of Aristotle, who points out that Melissus thinks he has a right to say that if that which has come into being has a beginning, that that which has not come into being has no beginning. Despite the fallaciousness of his reasoning, we can conjecture why it was that Melissus felt he must depart from Parmenides on the matter of the finitude of being. If being were a finite sphere, one could easily imagine that the sphere was bounded by the void, which could sound very much like saying that nothing is, what makes being what it is. The fragment just quoted seems to fluctuate between talk of a beginning in time and a spatial beginning; Melissus wants to deny both of being. It is eternal in duration and infinite in extension. This infinite extension is of a very curious kind, apparently, since Melissus also denies that being can be a body. "If being is, it must be one; and being one, it must have no body. If it were to have bulk, it would have parts and be no longer one." (Fr. 9) Tannery held that Melissus had arrived at the conception of immaterial being, but that this is not unequivocally so appears from the way in which Melissus speaks of the infinity of being. It had no beginning in time; but as well it has no limits as to spatial extension. It seems one thing to say that something is not spatially limited and that the notion of spatial limitation or illimitation is inapplicable to it. Fragment 9 is surely difficult to reconcile with Fragment 2, and we must admire Melissus' grasp of what seems to have escaped Parmenides, namely, that his spherical being despite his protestation, is divisible.
If we permit ourselves a few summary remarks on the Eleatic school, it is because something of extreme importance seems almost to have been reached by its members -- though doubtless for the wrong reasons. The sharp dichotomy drawn between reason and the senses is destined to have a great impact on subsequent philosophy, together with the allied implication that the object of reason must be immutable if there is to be a foundation for true knowledge. Whatever the senses report, change seems impossible for, if we admit it, we seem committed to the view that something comes from nothing. Better, then, to reject sense perception and accept only what makes sense to reason. This leads to extremely Pickwickian statements about being. With Parmenides we are faced with a unique, homogeneous sphere devoid of all sense qualities. Zeno defends this conception against attack by taking the opposed position and showing that it involves contradictory consequences. One might say that Zeno leaves the enemy demolished but the victory meaningless -- a recognition that has led to the description of Zeno as a sceptic for whom nothing is true, since all positions are susceptible of fatal attack. Whatever the truth of this description, Zeno is engaged solely in attacking the opposition and does not directly defend the position of Parmenides. Melissus, on the other hand, makes the Parmenidean doctrine his own, altering it when he feels that it is in need of alterations, lest it become an untenable position. Hence his great correction of the master when he says that being must be infinite. Further, being cannot be a body, since this would involve having parts. Though none of this leads to a definitely established grasp of the existence of being other than corporeal being, the language in which these men express themselves is such that what they say, as Aristotle pointed out, seems to have application beyond the physical world -- if there is such a beyond; for surely this is not evident. Doubtless it is anachronistic to speak of Parmenides as the first metaphysician but equally doubtless he has managed to present his thought in such a way that later thinkers saw a path opening before them which they were the first to trod. Another extremely important aspect of the Parmenidean school, particularly in Zeno, is its awareness of the force of the form of argumentation. An ideal is thereby set for future philosophizing and, consequently, a need gradually becomes recognized of examining for their own sake the forms of argument. It may be that when Aristotle called Zeno the father of dialectic, he meant dialectic in the sense of his own Topics, such that Zeno is seen to take the position granted him and to proceed from that. Nonetheless, Zeno can be called the father -- or at least the grandfather -- of logic in the wide sense, in that his rigorous procedure made men conscious of their own procedures in establishing the desired conclusion. Of more immediate impact was the doubt cast on the possibility of change and motion. If the admission of change involved the admission of something coming from nothing, then natural philosophy was in an impossible quandry. No one who wanted to continue the kind of speculation that had begun with the Ionians could conscientiously avoid the Parmenidean attack on motion and change. It is from this vantage point that we can best appreciate the efforts of Empedocles and Anaxagoras.
{17} Pythagoras is said to have coined the term "philosophy." See Kirk and Raven, p.229.
{18} See F. M. Cornford, Plato and Parminides (New York: Liberal Arts Press, 1957), pp. 1-27.