"… the person who seeks God discovers certain ways of coming to know him. These are also called proofs for the existence of God, not in the sense of proofs in the natural sciences, but rather in the sense of `converging and convincing arguments,' which allow us to attain certainty about the truth…

"…[from] The world: starting from movement, becoming, contingency, and the world's order and beauty, one can come to a knowledge of God as the origin and the end of the universe."[1]

As a mere "Peeping Thomist", I want to examine causes of motion, in the "physical" sense assumed by natural philosophy in the application of St. Thomas's Prima Via, which he regarded as the most manifest proof for the existence of God[4]. I probably offend many philosophers of science and Thomists, since my dialectic stumbles about within the realm of mobile being. I'll fumble beyond general, yet certain, notions of how motion is caused and seek its most proximate avenues.

How can we proceed? If any of Aristotle's insights into nature are still valid, they must be grounded on accessible "data". In analogy to modern physics, philosophy of nature has to presume a "correspondence principle". At the beginning of quantum mechanics Niels Bohr put forth the "correspondence principle" as a constraint on any new theory explaining microscopic phenomena: "Any new theory in physics … must reduce to a well established classical theory to which it corresponds when … applied under circumstances in which the less general theory is known to hold." So quantum mechanics applied to a macroscopic falling body must give the same predictions as Newton's Laws in the limit as the mass becomes macroscopic. We have a different cosmography than Aristotle's, but the "data" still must support his notions of nature in a post-Ptolemaic universe.

Presumably, according to Aristotelian/Thomistic principles, what is prior in intelligibility is necessary to understand what is subsequent. But first in priority, in relation to us, is motion. Aristotle's Physics was a science of mobile being as mobile with motion an attribute of mobile beings. Does modern physics presume such a simple idea? Perhaps. Contemporary physics texts can make it difficult for the beginning student to discern what the subject is about. My freshman text's first chapter began: "The building blocks of physics are the physical quantities we use to express the laws of physics. Among these are length, mass, time, force, velocity,…, resistivity,… and many more".[5] This very pragmatic "recipe" approach is typical. Another book begins with a final cause: "Why study physics? For two reasons. First, physics is one of the most fundamental of the sciences. Scientists of all disciplines make use of the ideas of physics, from chemists who study the structure of molecules to paleontologists who try to reconstruct how dinosaurs walked. Physics is also the foundation of all engineering and technology…But there's another reason. The study of physics is an adventure…It will appeal to your sense of beauty as well as your rational intelligence."[6] The dim voice of a perplexed freshman asks the simple question: "What is it about things that physics is trying to understand?" Listen to J. Richard Christman in "A Student's Companion to Physics" (an optional cheaper lighter book telling you more directly what the required expensive heavier book is about):

"Wherever we look, from the sub-microscopic world of fundamental particles to the grand scale of galaxies, we see objects in motion, influencing the motions of other objects … Mechanics is the study of motion. The goal is to understand exactly what aspects of the motion of one object are changed by the presence of other objects and exactly what properties objects must have in order to influence each other. The fundamental problem of mechanics is: given the relevant properties of a group of interacting objects, what are their motions?"

Take, for example, Newton's laws as applied to the simple harmonic oscillator problem (a block attached to a spring). The position and velocity of a moving mass at a time in the arbitrarily near future are deducible in terms of the current position and velocity. The force law describes how force is related to position. Newton's second law relates acceleration (a kinematic quantity) to force (identified with the pushes and pulls of ordinary experience). Hooke's Law describes the restoring force of an ideal spring: F = kx. The displacement x is a formal condition necessary for the spring to act with a value of force F. The spring constant k derives from the material conditions of the spring at the microscopic level. The minus sign indicates the restoring force tends to keep the mass in the vicinity of fixed position. The fact of the motion is implicit prior to the application of the dynamical laws because of conditions placed on the motion not directly related to Newton's second law, e.g. kinematic constraints. Any physics problem is resolved into a set of mathematical propositions including Newton's laws and kinematic constraints which can then deductively find the answer.

This becomes more evident in advanced formulations of classical mechanics by Lagrange, Hamilton, Jacoby, and others. Here, Newton's second law for each independent motion is drawn into a single unity insofar as all of the equations of motion for a system can be derived from a single scalar relation. First, one defines the total kinetic and potential energy functions of the system. The kinetic energy characterizes motions in the system. The potential energy characterizes positions and orientations. The "Lagrangian" function is constructed, which is the difference between the total kinetic and potential energies: L = T V. Now, suppose that we know the configurations of all the objects at a given initial time, t₁. What will the positions, velocities, and orientations be at a later time t₂? The system is imagined to follow a myriad of possible paths from time t₁ to t₂ and as it does the value of the Lagrangian function changes. The actual motions minimize a single quantity called the "action", defined as the time integral of the Lagrangian:

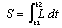
Least action techniques transfer to quantum mechanics and relativity theory giving crucial insights into the structure of mathematical physical laws. The equations of motion follow from minimizing the action integral, which itself depends on the Lagrangian. But can you "fiddle" with the Lagrangian and still get the correct equations of motion? By asking these kinds of questions Emmy Noether showed in a widely used theorem that certain properties of the system must be present.[8] For instance, we expect that when I perform a table-top experiment here and then perform it one kilometer away I should get the same results. This means the Lagrangian cannot depend explicitly on the position of where the experiment is performed. This independence of position gives rise to a symmetry that leads to a conserved quantity identified as momentum. This indifference to location is called the "homogeneity of space". If we require the same experiment to give the same results today as it did yesterday then the Lagrangian cannot depend explicitly on the time. This gives rise to a certain conserved quantity identified as the energy. Again, we might demand the experiment not depend on the orientation of my apparatus. This gives rise to conservation of angular momentum and the "isotropy of space". Surprising things are done in particle physics with Noether's theorem, with "gauge invariance" for example. By requiring that we cannot in anyway measure the phase of a charged particle wave function one arrives at the necessity for the electromagnetic field to be included in the correct Lagrangian.[9]

Thus, if modern physics presupposes bodies in motion, definable in Aristotle's terms,[10] natural philosophy claims there must be operative causes for motion. The most interesting expression of this appears in Book VII of Aristotle's Physics: "Whatever is moved is moved by another". This is the most problematic proposition of natural philosophy to establish in the present day in light of Newton's First Law of Motion,[11,12 ]and perhaps more so because of quantum mechanics. Curiously, as pointed out many times, Aristotle's law of efficient causality is not an a priori principle but is demonstrated based on the divisibility of motion. [13,14,15] The argument depends on Aristotle's conception of the continuum and how it applies to extended bodies, which are the foundation of the continuous nature of motion which then gives rise to the continuous nature of time. As far as I can tell, if natural philosophy does not validate this proposition in the way described, there is no hope of proving the existence of trans-mobile being from a consideration of mobile being. For now, we will take this proposition as established, as well as the subsequent proposition that "Mobile objects and motions cannot proceed to infinity", which establishes a per se order of moved movers giving the motion to a mobile being. One then draws the immediate conclusion: There must be an immobile First Mover.

Related to the discovery of the First Mover is how motion becomes implemented in the world which should bear some relation to physics and astronomy. Historically, astronomy sought insights into the agency of celestial motion as Fr. James Weisheipl pointed out in his article "The Celestial Movers in Medieval Physics."[16] "Mathematical Astronomy" was a mixed or "middle science" formally dependent upon mathematics for scientific validity but materially concerned with celestial bodies. Propter quid mathematical principles served to demonstrate scientific conclusions. "Physical Astronomy" used the mathematical methods as a tool to discover the real causes behind the quantity and so clarify the activity of the First Mover by identifying the order of proximate, particular, and universal causes of motion. Motion as a general property was caused ultimately by the First Mover acting on the first moved body, the outer celestial sphere. It was diversified instrumentally through other celestial bodies and the elements. A three-dimensional universe bounded by an outer two-dimensional surface configured into a one-dimensional series of concentric and subordinated moved-movers lent itself to such identifications. St. Thomas's great interest in all of this is evident by examining Summae and treatise articles on providence and governance in the world: "Whether God can move a body immediately?"[17] ; "Whether bodies obey the angels as regards local motion?"[18] ; "Whether the Heavenly Bodies Are the Cause of What is produced in bodies here below?"[19]

Oliva Blanchette underscored for me the different types of nature operative in the ancient cosmography when he drew my attention to "the radical and primordial meaning of `nature'."[20] Aristotle and St. Thomas distinguish things that have "mere" natural activity and those with something added in that "Mere nature implies only an order to place, and in bodies below the heavens, it implies order to one place only." Blanchette quotes St. Thomas from his commentary on De Caelo, "Of those things that are according to nature, some are bodies and magnitudes, like rocks and inanimate things, some have body and magnitude, like plants and animals, whose principle part is the soul (hence they are more what they are according to soul than according to body)".

Aristotle identified two radically distinct and irreducible realms based, in part, on the physical reality evident to us: motion[21,22] In the terrestrial region were the "up" and "down" rectilinear motions of the four elements radially oriented about the center of the universe. Circling above were celestial bodies with a motion without terminus and ever present. Aristotle defined nature as an intrinsic principle of motion in that to which it belongs primarily and not accidentally. Insight into nature is sought to provide a causal basis for why motion occurs. The mode of the nature acts as a middle term in demonstrating the why of the motion[23]. The natures of the four elements (earth, water, air, fire), were part of the answer to why they moved to determinate places. Their places were deemed "proper" since they characterized the bodies in question. Since celestial bodies did not move toward a determinant place, they did not have an active intrinsic principle characteristic of their type. St. Thomas described their mode of nature saying "… a celestial body, considered in its substance, is found to be indifferently related to every place, just as prime matter is to every form... Of course, it is a different situation in the case of a heavy and light body which, considered in its nature, is not indifferent to a place of its own. So, the nature of a heavy or light body is the active principle of its motion, while the nature of a celestial body is the passive principle of its motion" (see especially St. Thomas Aquinas, Summa Contra Gentiles, Bk 3, Pt. I, Q. 23, [8-11]).

Even after the dust has settled from the Copernican revolution we still observe celestial bodies moving with a motion without a terminating place. In this sense, a groping attempt to explain the "naturalness" of their motion might emulate the mode Aquinas used to explain them. Remembering our correspondence principle: similar types of motions give similar insights into the mode of nature. Aristotle was mistaken in identifying the seat of the diurnal celestial motion as the sphere of fixed stars, it is now known to be the daily rotation of the earth. This observed geocentric motion was identified to be passive in its natural character because it does not seek a determinate place. Thus, we might now say the nature of the rotating earth is passive insofar as it does not seek a determinate mode of rest. The active modes of natural activity we observe are embedded or contained within a common passive mode, just as was observed by Aristotle.

To be sure, modern physics does presume natural things as having active principles. Newtonian mechanics by itself can obscure naturalistic description. Vincent Smith pointed out the example of a boy pulling a sled on snow. There is nothing in the mechanical problem that would indicate the boy originates the motion, in a way the sled does not. Thermodynamics grasps something of this through the first law of thermodynamics, , which is not derivable from the mechanical laws.[24] The source of energy for the motion of the system, (boy + sled), comes from the internal energy of the boy and speaks to a priority of the boy as a principle. The standard model of particle physics relates all known activity of matter to manifestations of quarks, leptons, and gauge bosons.[25] For example, the electromagnetic force is seen as a manifestation of the exchange of virtual photons between particles with electric charge. The strong nuclear force is seen as an exchange of virtual gluons between quark particles. The vertices of Feynman diagrams, where virtual particles affect the four-momentum states of real particles, express proximate natural activity at a fundamental level. There is a capacity for a charged particle to emit virtual photons, in violation of energy conservation, as long as the debt is paid back within a time granted by the Heisenberg uncertainty principle. The cumulative affect of these exchanges results in accelerations which we infer to be a "force". The boson fields are somehow instrumental for the activity of the charged particles which themselves have a receptivity with respect to the field to receive the impressions of neighboring charges. Even with the principle of super-position for wave functions, the interactions represented in the components of the wave function depend upon the motion of the virtual photons which in turn influence the motion of charged particles. What is called the "vacuum" is anything but the ontological void described by Aristotle as a "place without an occupying body". Virtual particles presumably "cannot live an existence independent of the charges that emit or absorb them."[26] But, at least conceptually, these transient objects are thought of as "propagating" for a certain period of time and so it is implied they have a kind of motion that is inertial from the time of emission to the time of absorption.

There are at least two modes by which objects can be seen as moving passively. These modes follow from the homegeneity and isotropy of space mentioned with respect to Noether's theorem and it's lack of local preferences in the application of physical laws. They are motion at a constant rate along a fixed direction in the absence of external forces (Law of Inertia; conservation of linear momentum) and rotation at a constant rate about a fixed direction in the absence of external torques (conservation of angular momentum).

How do such passive motions arise? Do they result from the activity of material objects? Force laws have a compelling character because certain aspects of motion for a particular body (i.e. accelerations) are related to other bodies that are more or less proximate. Even the fields mediating interactions have quantitative determinations possessing motion (i.e. electromagnetic waves). Newton's laws of motion (and later generalizations in special relativity) are valid in inertial frames of reference. When one observes a system in a frame of reference that is not inertial it introduces "inertial" (some say "fictitious") forces required to relate observed kinematics to the dynamical laws. Inertial forces are environmental but cannot be localized to any particular body as such, except as relating to motion in another inertial frame. They are not action-reaction pair forces. Their proportionality to mass makes them similar to gravity in how they act and gave Einstein inspiration for the "elevator" thought experiment through which he established the equivalence principle.

Influencing Einstein in formulating General Relativity was the hope that it could explain the origin of inertial motion by Mach's principle. Ernst Mach presented the idea in The Science of Mechanics that it is not reasonable to speak of accelerations relative to an absolute space over and above material bodies. He conjectured that the natural candidate for the frame of reference defining inertial motions would be that which is at rest with respect to distant stars. As developed by Einstein and others the motions of material bodies are influenced by the large scale distribution of matter, subject to a number of specific conditions. As summarized in the standard text Gravitation by Misner, Thorne, and Wheeler these conditions are: First the universe must have a large scale geometry that is "closed", finite in spatial extent and volume with no boundaries or edges. The metric geometry of space along a fixed space-like hyper-surface must be specified and in self-consistent accord with the mass-energy densities of all non-gravitational fields through the Einstein field equations. With a few other technical details added, one then can use the remaining components of the Einstein field equations to determine the 4-geometry of the universe extending into the past and future. In their words, "In this way, the inertial properties of every test particle are determined everywhere and at all times, giving concrete realization of Mach's principle."[27] The interpretation invoked here is that inertial motion is a manifestation of the geometry of space-time since the local geometry is affected by the presence of matter everywhere else in the universe.

This central insight is elaborated by Ciufolini and Wheeler in the recent work Gravitation and Inertia,

"Inertia here, in the sense of local inertial frames, that is the grip of spacetime here on mass here, is fully defined by the geometry, the curvature, the structure of spacetime here. The geometry here, however, has to fit smoothly to the geometry of the immediate surroundings; those domains, onto their surroundings; and so on, all the way around the great curve of space. Moreover, the geometry in each local region responds in its curvature to the mass in that region. Therefore, every bit of momentum-energy, wherever located, makes its influence felt on the geometry of space throughout the whole universe - and felt, thus, on inertia right here.

"…In other words, inertia (local inertial frames) everywhere and at all times is totally fixed, specified, determined, by the initial distribution of momentum-energy, of mass and mass-in-motion. The mathematics cries out with all the force at its command that mass there does determine inertia here."[28]

Does such a General Relativistic proscription completely account for inertia? To determine the free-falling inertial frames that we call gravitation, the mass-energy-momentum stress tensor must be specified at every location of space-time. To understand the components of that tensor we must conceive the matter in the universe as in motion. At best, this kind of model for the universe does not explain motion, as such, in the causal way aspired to by natural philosphy, but states a formal self-consistency relation. General Relativity is constructed so that within each local region of space-time, if taken to be sufficiently small, one can always find a frame of reference which is locally inertial. These local inertial frames can be found in any coordinate system by the geodesic equation. Along these free-falling local frames, inertial motion is possible at least over short times. So we might say that this implementation of Mach's principle determines what local frames will be inertial but does not account for the fact they are there to begin with. Steven Weinberg spoke of these limitations when he wrote:

"In the absence of nearby matter, the inertial frames are determined by the mean cosmic gravitational field, which in turn is determined by the mean mass density of stars, so it is not surprising that their inertial frames are at rest, or in a state of uniform non-rotating motion, with respect to the typical stars. When a large mass like the sun is brought close, it changes the inertial frames so that they accelerate toward the mass, but the laws of motion in these freely falling frames are still the laws of special relativity, and show no effects of the surrounding mass distribution. In this sense, the equivalence principle and Mach's principle are in direct opposition."[31]